U. Rovira i Virgili

This is the email communication network at the University Rovira i Virgili in Tarragona in the south of Catalonia in Spain. Nodes are users and each edge represents that at least one email was sent. The direction of emails and the number of emails between two persons are not stored.

Metadata

Code		`A@`
Internal name		`arenas-email`
Name		U. Rovira i Virgili
Data source		http://deim.urv.cat/~aarenas/data/welcome.htm
Availability		Dataset is available for download
Consistency check		Dataset passed all tests
Category		Communication network
Node meaning		User
Edge meaning		Communication
Network format		Unipartite, undirected
Edge type		Unweighted, no multiple edges
Loops		Does not contain loops
Orientation		Is not directed, but the underlying data is
Multiplicity		Does not have multiple edges, but the underlying data has

Statistics

Size	n =	1,133
Volume	m =	5,451
Loop count	l =	0
Wedge count	s =	96,415
Claw count	z =	817,974
Cross count	x =	6,550,542
Triangle count	t =	5,343
Square count	q =	43,591
4-Tour count	T₄ =	745,290
Maximum degree	d_max =	71
Average degree	d =	9.622 24
Fill	p =	0.008 500 21
Size of LCC	N =	1,133
Diameter	δ =	8
50-Percentile effective diameter	δ_0.5 =	3.179 35
90-Percentile effective diameter	δ_0.9 =	4.475 95
Median distance	δ_M =	4
Mean distance	δ_m =	3.654 76
Gini coefficient	G =	0.491 113
Balanced inequality ratio	P =	0.314 529
Relative edge distribution entropy	H_er =	0.942 894
Power law exponent	γ =	1.561 09
Tail power law exponent	γ_t =	6.771 00
Tail power law exponent with p	γ₃ =	6.771 00
p-value	p =	0.752 000
Degree assortativity	ρ =	+0.078 201 0
Degree assortativity p-value	p_ρ =	2.915 87 × 10⁻¹⁶
Clustering coefficient	c =	0.166 250
Spectral norm	α =	20.747 0
Algebraic connectivity	a =	0.332 560
Spectral separation	\|λ₁[A] / λ₂[A]\| =	1.223 03
Non-bipartivity	b_A =	0.592 240
Normalized non-bipartivity	b_N =	0.235 829
Algebraic non-bipartivity	χ =	0.334 159
Spectral bipartite frustration	b_K =	0.008 681 95
Controllability	C =	60
Relative controllability	C_r =	0.052 956 8

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

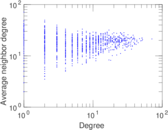
Matrix decompositions plots

Downloads

References

[1]	Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2]	Roger Guimerà, Leon Danon, Albert Díaz-Guilera, Francesc Giralt, and Alex Arenas. Self-similar community structure in a network of human interactions. Phys. Rev. E, 68(6):065103, 2003.