Amazon (MDS)

This is the co-purchase network of Amazon based on the "customers who bought this also bought" feature. Nodes are products and an undirected edge between two nodes shows that the corresponding products have been frequently bought together.

Metadata

CodeCA
Internal namecom-amazon
NameAmazon (MDS)
Data sourcehttp://snap.stanford.edu/data/com-Amazon.html
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Miscellaneous network
Node meaningProduct
Edge meaningCo-purchase
Network formatUnipartite, undirected
Edge typeUnweighted, no multiple edges
LoopsDoes not contain loops

Statistics

Size n =334,863
Volume m =925,872
Loop count l =0
Wedge count s =9,752,186
Claw count z =142,823,893
Cross count x =6,722,504,872
Triangle count t =667,129
Square count q =3,125,323
4-Tour count T4 =65,863,072
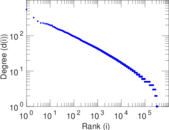
Maximum degree dmax =549
Average degree d =5.529 86
Fill p =1.651 38 × 10−5
Size of LCC N =334,863
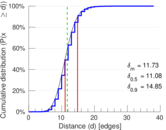
Diameter δ =47
50-Percentile effective diameter δ0.5 =11.076 0
90-Percentile effective diameter δ0.9 =14.851 4
Median distance δM =12
Mean distance δm =11.725 3
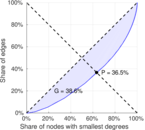
Gini coefficient G =0.385 905
Balanced inequality ratio P =0.364 978
Relative edge distribution entropy Her =0.976 681
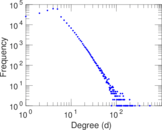
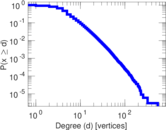
Power law exponent γ =1.691 22
Tail power law exponent γt =3.581 00
Tail power law exponent with p γ3 =3.581 00
p-value p =0.023 000 0
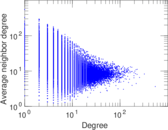
Degree assortativity ρ =−0.058 819 6
Degree assortativity p-value pρ =0.000 00
Clustering coefficient c =0.205 224
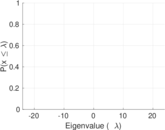
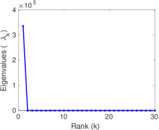
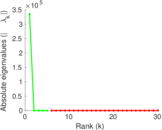
Spectral norm α =23.975 6
Algebraic connectivity a =0.000 586 130
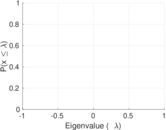
Spectral separation |λ1[A] / λ2[A]| =1.002 63
Non-bipartivity bA =0.031 835 0
Normalized non-bipartivity bN =0.012 895 0
Algebraic non-bipartivity χ =0.026 216 2
Spectral bipartite frustration bK =0.001 185 21
Controllability C =45,973
Relative controllability Cr =0.137 289

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Delaunay graph drawing

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

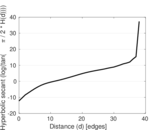
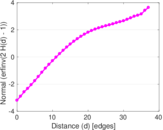
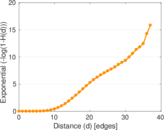
Matrix decompositions plots

Downloads

References

[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] Jaewon Yang and Jure Leskovec. Defining and evaluating network communities based on ground-truth. In Proc. ACM SIGKDD Workshop on Min. Data Semant., page 3, 2012.