Condensed matter (2005)

These are co-authorships between authors of papers uploaded to the "condensed matter" section of arXiv. As noted from the dataset source: "[...] the original graph contains two edges with weight inf[ormation], these were removed before making the graph unweighted."

Metadata

CodeCM2
Internal namedimacs10-cond-mat-2005
NameCondensed matter (2005)
Data sourcehttps://www.cc.gatech.edu/dimacs10/archive/clustering.shtml
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Co-authorship network
Dataset timestamp 1995-01-01 ⋯ 2005-03-31
Node meaningAuthor
Edge meaningCo-authorship
Network formatUnipartite, undirected
Edge typeUnweighted, no multiple edges
LoopsDoes not contain loops
Join Is the join of an underlying network
Multiplicity Does not have multiple edges, but the underlying data has

Statistics

Size n =39,577
Volume m =175,691
Loop count l =0
Wedge count s =4,629,240
Claw count z =106,646,864
Cross count x =3,241,514,847
Triangle count t =378,059
Square count q =4,325,174
4-Tour count T4 =53,469,734
Maximum degree dmax =278
Average degree d =8.878 44
Fill p =0.000 224 339
Size of LCC N =36,458
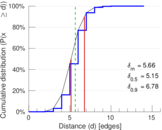
Diameter δ =18
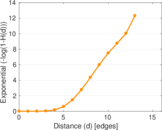
50-Percentile effective diameter δ0.5 =5.145 42
90-Percentile effective diameter δ0.9 =6.777 24
Median distance δM =6
Mean distance δm =5.658 82
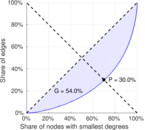
Gini coefficient G =0.540 149
Balanced inequality ratio P =0.299 617
Relative edge distribution entropy Her =0.947 144
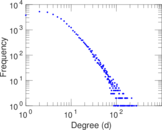
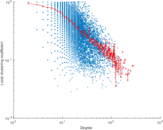
Power law exponent γ =1.602 16
Tail power law exponent γt =3.651 00
Tail power law exponent with p γ3 =3.651 00
p-value p =0.147 000
Degree assortativity ρ =+0.186 327
Degree assortativity p-value pρ =0.000 00
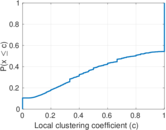
Clustering coefficient c =0.245 003
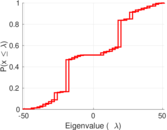
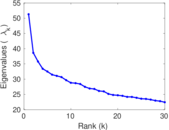
Spectral norm α =51.285 7
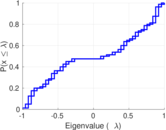
Algebraic connectivity a =0.019 318 5
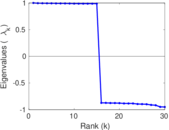
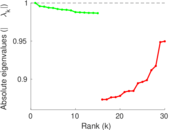
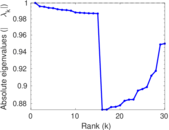
Spectral separation |λ1[A] / λ2[A]| =1.327 44
Non-bipartivity bA =0.641 674
Normalized non-bipartivity bN =0.050 332 4
Algebraic non-bipartivity χ =0.088 251 8
Spectral bipartite frustration bK =0.002 341 91
Controllability C =2,467
Relative controllability Cr =0.062 334 2

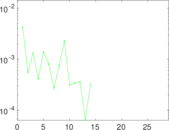
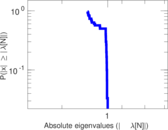
Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Delaunay graph drawing

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

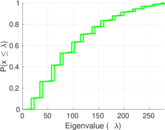
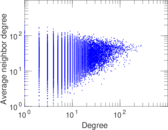
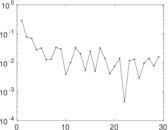
Matrix decompositions plots

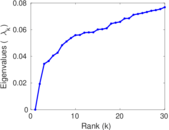
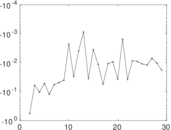
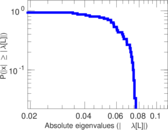
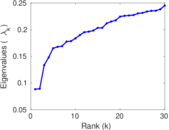
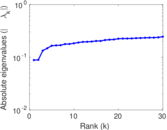
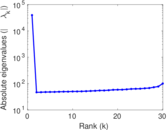
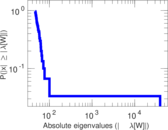
Downloads

References

[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] Mark E. J. Newman. The structure of scientific collaboration networks. Proc. Natl. Acad. Sci. U.S.A., 98(2):404–409, 2001.