Network science

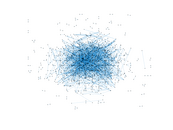
This is a network of co-authorships in the area of network science.

Metadata

Code		`NS`
Internal name		`dimacs10-netscience`
Name		Network science
Data source		https://www.cc.gatech.edu/dimacs10/archive/clustering.shtml
Availability		Dataset is available for download
Consistency check		Dataset passed all tests
Category		Co-authorship network
Dataset timestamp		2006
Node meaning		Author
Edge meaning		Co-authorship
Network format		Unipartite, undirected
Edge type		Unweighted, no multiple edges
Loops		Does not contain loops
Join		Is the join of an underlying network
Multiplicity		Does not have multiple edges, but the underlying data has

Size	n =	1,461
Volume	m =	2,742
Loop count	l =	0
Wedge count	s =	16,284
Claw count	z =	57,925
Cross count	x =	221,122
Triangle count	t =	3,764
Square count	q =	22,787
4-Tour count	T₄ =	252,916
Maximum degree	d_max =	34
Average degree	d =	3.753 59
Fill	p =	0.002 570 95
Size of LCC	N =	379
Diameter	δ =	17
50-Percentile effective diameter	δ_0.5 =	5.642 87
90-Percentile effective diameter	δ_0.9 =	9.182 06
Median distance	δ_M =	6
Mean distance	δ_m =	6.285 28
Gini coefficient	G =	0.415 264
Balanced inequality ratio	P =	0.346 827
Relative edge distribution entropy	H_er =	0.957 949
Power law exponent	γ =	1.970 78
Tail power law exponent	γ_t =	3.611 00
Tail power law exponent with p	γ₃ =	3.611 00
p-value	p =	0.004 000 00
Degree assortativity	ρ =	+0.461 622
Degree assortativity p-value	p_ρ =	1.231 55 × 10⁻²⁸⁷
Clustering coefficient	c =	0.693 441
Spectral norm	α =	19.023 8
Algebraic connectivity	a =	0.015 203 8
Spectral separation	\|λ₁[A] / λ₂[A]\| =	1.833 54
Non-bipartivity	b_A =	0.712 488
Normalized non-bipartivity	b_N =	0.255 201
Algebraic non-bipartivity	χ =	0.462 123
Spectral bipartite frustration	b_K =	0.023 953 0
Controllability	C =	105
Relative controllability	C_r =	0.071 868 6

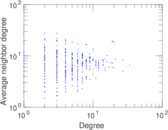
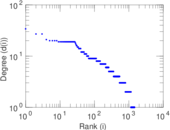
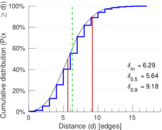
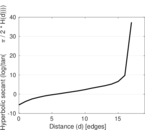
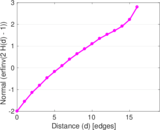
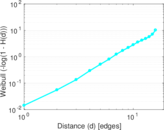
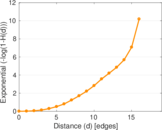
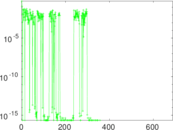
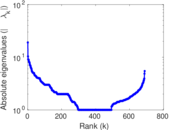
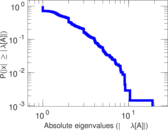
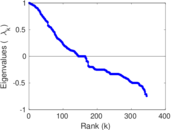
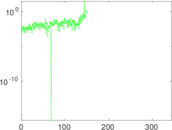
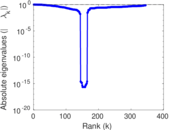
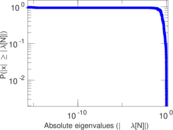
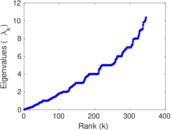
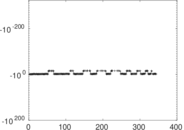
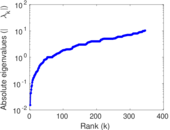
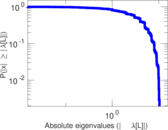
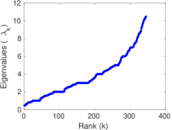
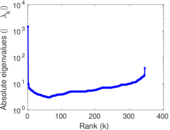
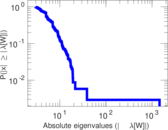
[1]	Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2]	Mark E. J. Newman. Finding community structure in networks using the eigenvectors of matrices. Phys. Rev. E, 74(3), 2006.