Edinburgh Associative Thesaurus
This is the Edinburgh Associative Thesaurus. Nodes are English words, and a
directed link from A to B denotes that the word B was given as a response to
the stimulus word A in user experiments. Multiple links are allowed, and the
multiplicity of an edge denotes the number of times a word has been given as a
response to another word. In some case, the given word itself was given as a
response, leading to loops in the network.
Metadata
Statistics
| Size | n = | 23,132
|
| Volume | m = | 511,764
|
| Unique edge count | m̿ = | 312,310
|
| Loop count | l = | 710
|
| Wedge count | s = | 30,388,904
|
| Claw count | z = | 2,967,734,991
|
| Cross count | x = | 376,129,003,421
|
| Triangle count | t = | 409,174
|
| Square count | q = | 16,682,968
|
| 4-Tour count | T4 = | 255,613,548
|
| Maximum degree | dmax = | 1,076
|
| Maximum outdegree | d+max = | 78
|
| Maximum indegree | d−max = | 1,044
|
| Average degree | d = | 44.247 3
|
| Fill | p = | 0.000 583 659
|
| Average edge multiplicity | m̃ = | 1.638 64
|
| Size of LCC | N = | 23,132
|
| Size of LSCC | Ns = | 7,751
|
| Relative size of LSCC | Nrs = | 0.335 077
|
| Diameter | δ = | 6
|
| 50-Percentile effective diameter | δ0.5 = | 2.935 81
|
| 90-Percentile effective diameter | δ0.9 = | 3.852 38
|
| Median distance | δM = | 3
|
| Mean distance | δm = | 3.431 44
|
| Gini coefficient | G = | 0.696 577
|
| Balanced inequality ratio | P = | 0.231 681
|
| Outdegree balanced inequality ratio | P+ = | 0.437 125
|
| Indegree balanced inequality ratio | P− = | 0.198 509
|
| Relative edge distribution entropy | Her = | 0.911 566
|
| Power law exponent | γ = | 1.469 67
|
| Tail power law exponent | γt = | 3.211 00
|
| Tail power law exponent with p | γ3 = | 3.211 00
|
| p-value | p = | 0.001 000 00
|
| Outdegree tail power law exponent with p | γ3,o = | 6.591 00
|
| Outdegree p-value | po = | 0.000 00
|
| Indegree tail power law exponent with p | γ3,i = | 1.731 00
|
| Indegree p-value | pi = | 0.000 00
|
| Degree assortativity | ρ = | −0.047 673 9
|
| Degree assortativity p-value | pρ = | 5.650 03 × 10−296
|
| In/outdegree correlation | ρ± = | +0.532 290
|
| Clustering coefficient | c = | 0.040 393 8
|
| Directed clustering coefficient | c± = | 0.051 384 5
|
| Spectral norm | α = | 189.271
|
| Operator 2-norm | ν = | 131.648
|
| Cyclic eigenvalue | π = | 57.307 7
|
| Algebraic connectivity | a = | 0.961 452
|
| Spectral separation | |λ1[A] / λ2[A]| = | 1.526 69
|
| Reciprocity | y = | 0.095 674 2
|
| Non-bipartivity | bA = | 0.542 479
|
| Normalized non-bipartivity | bN = | 0.482 007
|
| Algebraic non-bipartivity | χ = | 0.814 787
|
| Spectral bipartite frustration | bK = | 0.007 915 30
|
| Controllability | C = | 14,922
|
| Relative controllability | Cr = | 0.645 080
|
Plots

















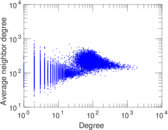

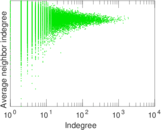























Matrix decompositions plots




























































































Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
George R. Kiss, Christine Armstrong, and Robert Milroy.
An associative thesaurus of English and its computer analysis.
The Comput. and Literary Studies, 1973.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee






































































































































