Flickr
This is the bipartite network of Flickr users and their group memberships.
Metadata
Statistics
| Size | n = | 499,610
|
| Left size | n1 = | 395,979
|
| Right size | n2 = | 103,631
|
| Volume | m = | 8,545,307
|
| Wedge count | s = | 9,755,612,929
|
| Claw count | z = | 34,392,804,882,987
|
| Cross count | x = | 183,897,960,553,802,528
|
| Square count | q = | 35,258,013,095
|
| 4-Tour count | T4 = | 321,104,865,090
|
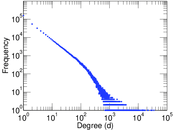
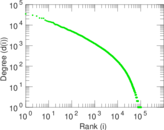
| Maximum degree | dmax = | 34,989
|
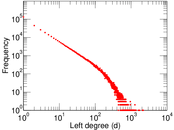
| Maximum left degree | d1max = | 2,186
|
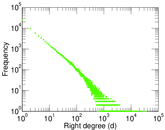
| Maximum right degree | d2max = | 34,989
|
| Average degree | d = | 34.207 9
|
| Average left degree | d1 = | 21.580 2
|
| Average right degree | d2 = | 82.459 0
|
| Fill | p = | 0.000 208 241
|
| Size of LCC | N = | 470,345
|
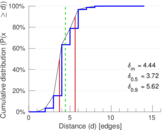
| Diameter | δ = | 18
|
| 50-Percentile effective diameter | δ0.5 = | 3.720 57
|
| 90-Percentile effective diameter | δ0.9 = | 5.622 14
|
| Median distance | δM = | 4
|
| Mean distance | δm = | 4.437 56
|
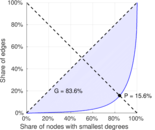
| Gini coefficient | G = | 0.849 060
|
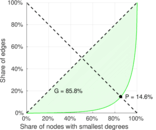
| Balanced inequality ratio | P = | 0.144 240
|
| Left balanced inequality ratio | P1 = | 0.178 314
|
| Right balanced inequality ratio | P2 = | 0.146 343
|
| Relative edge distribution entropy | Her = | 0.858 658
|
| Power law exponent | γ = | 1.583 20
|
| Tail power law exponent | γt = | 1.451 00
|
| Degree assortativity | ρ = | −0.052 003 7
|
| Degree assortativity p-value | pρ = | 0.000 00
|
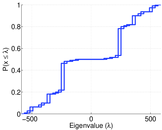
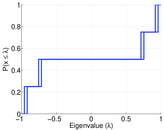
| Spectral norm | α = | 587.208
|
| Spectral separation | |λ1[A] / λ2[A]| = | 1.784 40
|
Plots
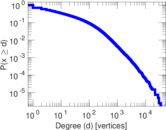
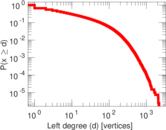
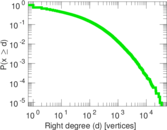
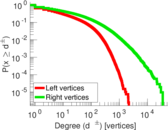






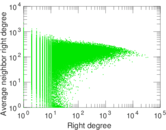
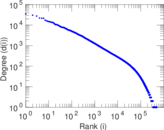
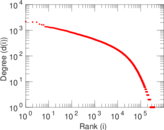

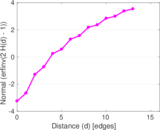
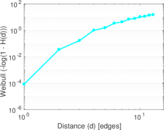
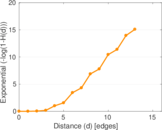
Matrix decompositions plots
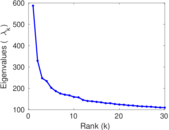
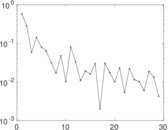
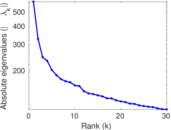
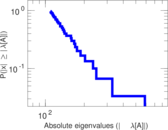
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Alan Mislove, Massimiliano Marcon, Krishna P. Gummadi, Peter Druschel, and
Bobby Bhattacharjee.
Measurement and analysis of online social networks.
In Proc. Internet Measurement Conf., 2007.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee