TREC (disks 4–5)

This is the bipartite network of 556,000 text documents from the Text Retrieval Conference's (TREC) Disks 4 and 5, containing 1.1 million words. Each edge represents one document-word inclusion.

Metadata

CodeTR
Internal namegottron-trec
NameTREC (disks 4–5)
Data sourcehttp://www.nist.gov/tac/data/data_desc.html#TREC
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Text network
Node meaningDocument, word
Edge meaningInclusion
Network formatBipartite, undirected
Edge typeUnweighted, multiple edges

Statistics

Size n =1,729,302
Left size n1 =556,077
Right size n2 =1,173,225
Volume m =151,632,178
Unique edge count m̿ =83,629,405
Wedge count s =1,604,790,310,718
Claw count z =58,090,382,597,882,208
Cross count x =3.122 × 1021
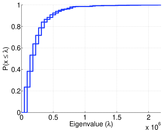
Maximum degree dmax =457,437
Maximum left degree d1max =30,701
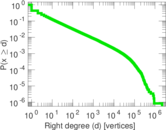
Maximum right degree d2max =457,437
Average degree d =175.368
Average left degree d1 =272.682
Average right degree d2 =129.244
Fill p =0.000 128 187
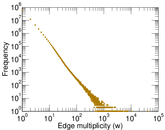
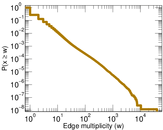
Average edge multiplicity m̃ =1.813 14
Size of LCC N =1,725,011
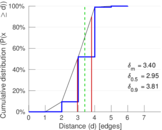
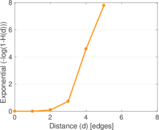
Diameter δ =7
50-Percentile effective diameter δ0.5 =2.953 78
90-Percentile effective diameter δ0.9 =3.808 95
Median distance δM =3
Mean distance δm =3.395 75
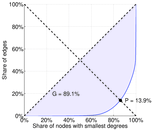
Gini coefficient G =0.854 045
Balanced inequality ratio P =0.168 945
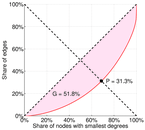
Left balanced inequality ratio P1 =0.312 897
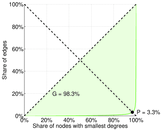
Right balanced inequality ratio P2 =0.033 487 1
Relative edge distribution entropy Her =0.809 386
Power law exponent γ =1.503 70
Tail power law exponent γt =1.401 00
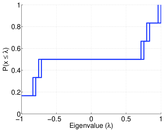
Degree assortativity ρ =−0.068 551 7
Degree assortativity p-value pρ =0.000 00
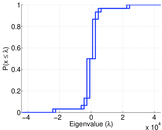
Spectral norm α =44,117.0

Plots

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the normalized adjacency matrix

Hop distribution

Edge weight/multiplicity distribution

Downloads

References

[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] National Institute of Standards and Technology. Text REtrieval Conference (TREC) English documents. http://trec.nist.gov/data/docs_eng.html, August 2010. Volume 4 & 5.