CiaoDVD trust
This is the user–user trust network of the site http://dvd.ciao.co.uk/ from
2013.
Metadata
Statistics
| Size | n = | 4,658
|
| Volume | m = | 40,133
|
| Loop count | l = | 0
|
| Wedge count | s = | 2,614,007
|
| Claw count | z = | 247,680,289
|
| Cross count | x = | 13,147,053,018
|
| Triangle count | t = | 156,501
|
| Square count | q = | 9,178,144
|
| 4-Tour count | T4 = | 83,947,412
|
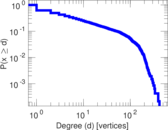
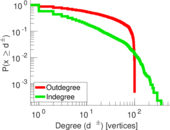
| Maximum degree | dmax = | 419
|
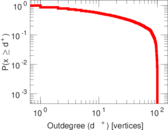
| Maximum outdegree | d+max = | 100
|
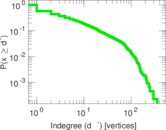
| Maximum indegree | d−max = | 361
|
| Average degree | d = | 17.231 9
|
| Fill | p = | 0.001 850 10
|
| Size of LCC | N = | 4,562
|
| Size of LSCC | Ns = | 1,032
|
| Relative size of LSCC | Nrs = | 0.221 554
|
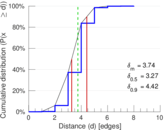
| Diameter | δ = | 9
|
| 50-Percentile effective diameter | δ0.5 = | 3.273 89
|
| 90-Percentile effective diameter | δ0.9 = | 4.421 73
|
| Median distance | δM = | 4
|
| Mean distance | δm = | 3.743 68
|
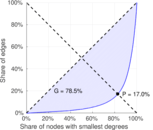
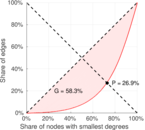
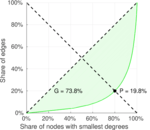
| Gini coefficient | G = | 0.785 007
|
| Balanced inequality ratio | P = | 0.170 458
|
| Outdegree balanced inequality ratio | P+ = | 0.269 429
|
| Indegree balanced inequality ratio | P− = | 0.198 141
|
| Relative edge distribution entropy | Her = | 0.844 275
|
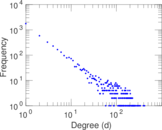
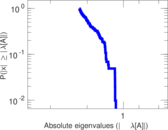
| Power law exponent | γ = | 1.745 98
|
| Tail power law exponent | γt = | 1.551 00
|
| Tail power law exponent with p | γ3 = | 1.551 00
|
| p-value | p = | 0.000 00
|
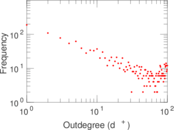
| Outdegree tail power law exponent with p | γ3,o = | 1.711 00
|
| Outdegree p-value | po = | 0.000 00
|
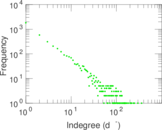
| Indegree tail power law exponent with p | γ3,i = | 1.631 00
|
| Indegree p-value | pi = | 0.000 00
|
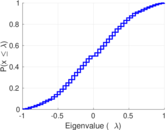
| Degree assortativity | ρ = | +0.103 916
|
| Degree assortativity p-value | pρ = | 2.136 28 × 10−158
|
| In/outdegree correlation | ρ± = | +0.551 072
|
| Clustering coefficient | c = | 0.179 610
|
| Directed clustering coefficient | c± = | 0.195 143
|
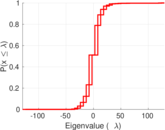
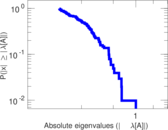
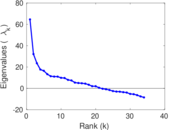
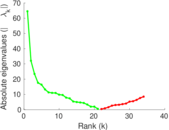
| Spectral norm | α = | 129.218
|
| Operator 2-norm | ν = | 70.147 7
|
| Cyclic eigenvalue | π = | 59.518 3
|
| Algebraic connectivity | a = | 0.107 634
|
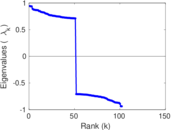
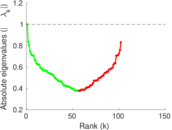
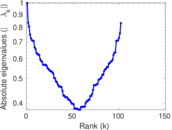
| Spectral separation | |λ1[A] / λ2[A]| = | 1.998 12
|
| Reciprocity | y = | 0.349 687
|
| Non-bipartivity | bA = | 0.790 358
|
| Normalized non-bipartivity | bN = | 0.062 702 3
|
| Algebraic non-bipartivity | χ = | 0.107 350
|
| Spectral bipartite frustration | bK = | 0.001 851 79
|
| Controllability | C = | 3,247
|
| Relative controllability | Cr = | 0.697 080
|
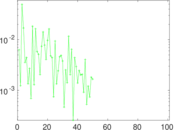
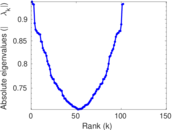
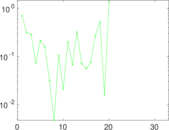
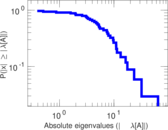
Plots

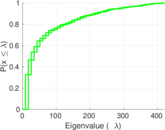
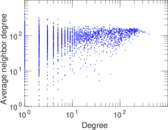

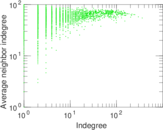
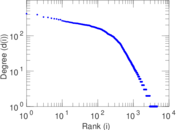

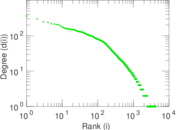
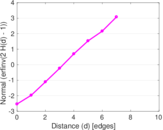
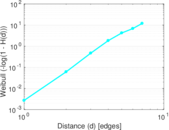
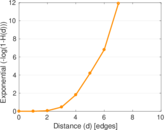




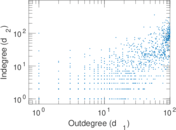
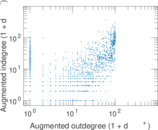
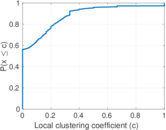
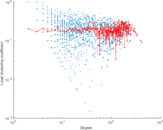
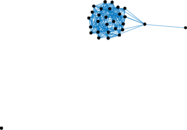
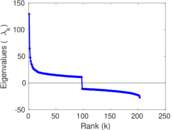
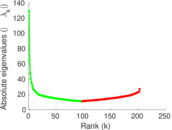
Matrix decompositions plots
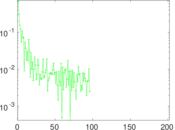
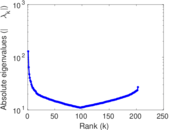


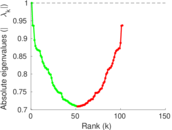
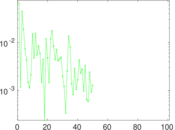
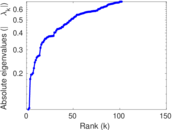
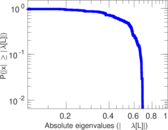
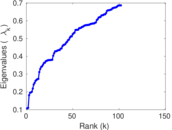

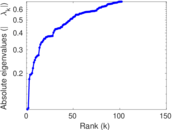

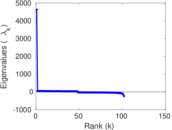
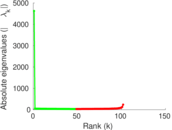
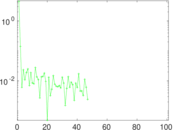
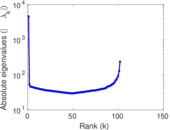
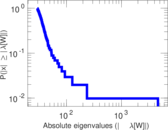
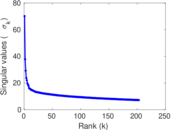
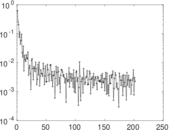
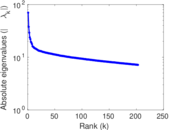
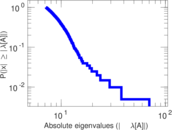
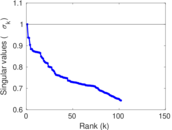
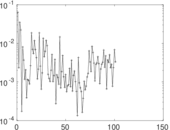
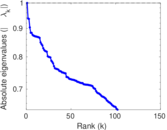

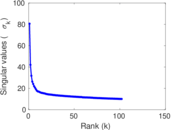
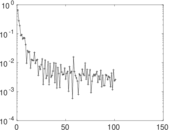
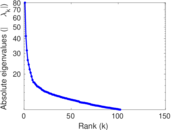

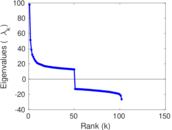
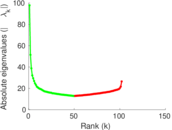
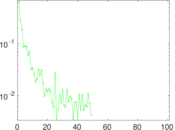
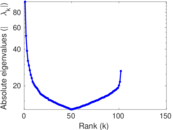
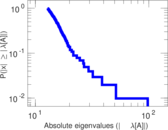

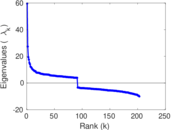
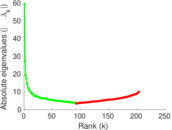
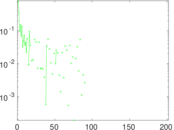

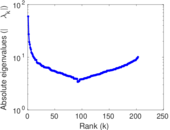
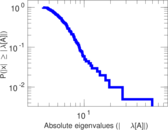
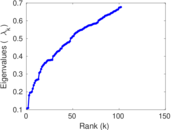
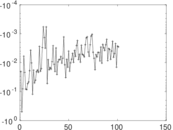
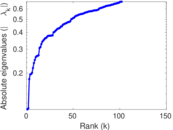

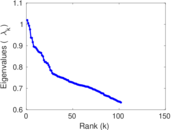
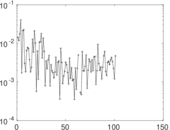
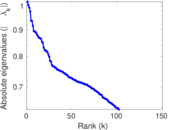
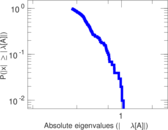

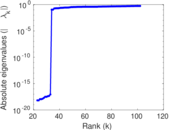
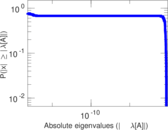
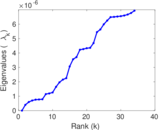
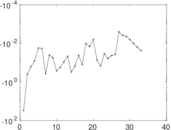
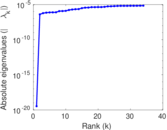
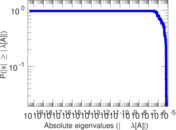
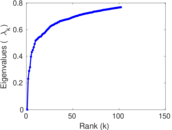
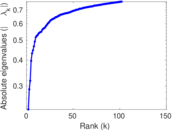

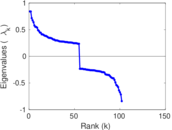
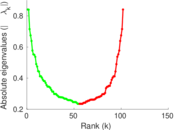

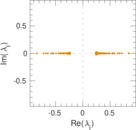
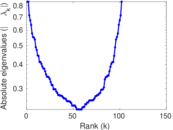
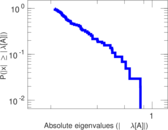
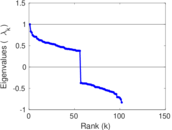

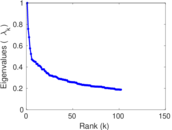
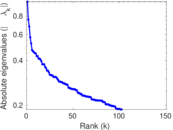
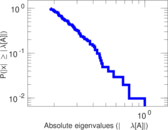


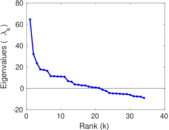
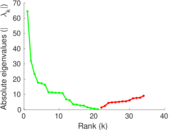
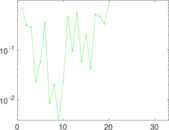
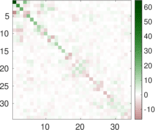
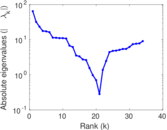
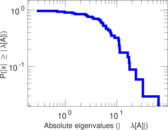
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Guobing Guo, Jie Zhang, Daniel Thalmann, and Neil Yorke-Smith.
ETAF: An extended trust antecedents framework for trust prediction.
In Proc. Int. Conf. Adv. in Soc. Netw. Anal. and Min., pages
540–547, 2014.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee















































































































































