Human proteins (Figeys)

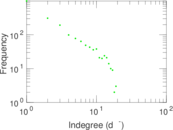
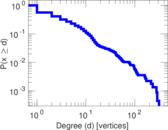
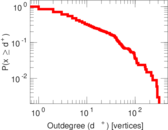
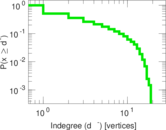
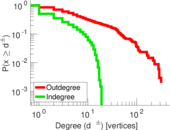
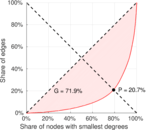
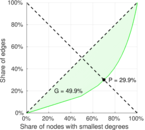
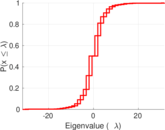
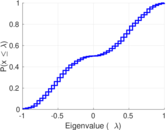
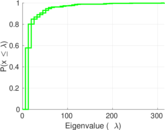
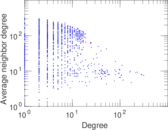
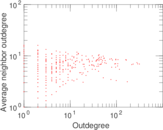
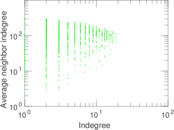
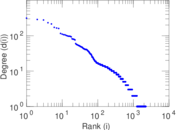
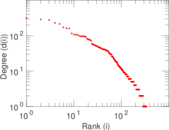
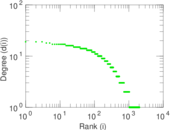
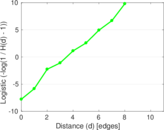
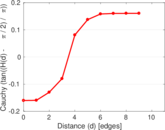
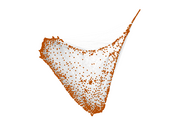
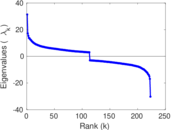
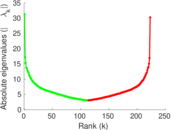
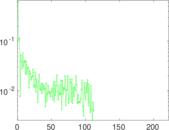
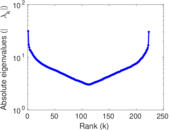
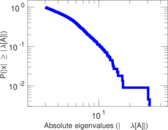
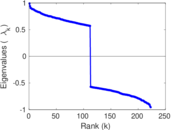
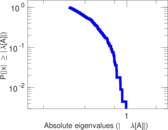
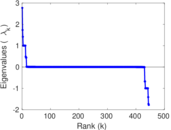
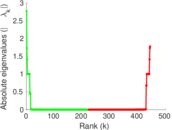
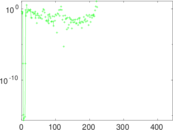
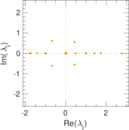
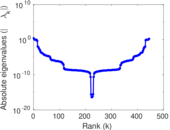
This is a network of interactions between proteins in Humans (Homo sapiens), from the first large-scale study of protein–protein interactions in Human cells using a mass spectrometry-based approach.

Metadata

Code		`MF`
Internal name		`maayan-figeys`
Name		Human proteins (Figeys)
Data source		http://research.mssm.edu/maayan/datasets/qualitative_networks.shtml
Availability		Dataset is available for download
Consistency check		Dataset passed all tests
Category		Metabolic network
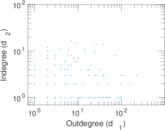
Node meaning		Protein
Edge meaning		Interaction
Network format		Unipartite, directed
Edge type		Unweighted, no multiple edges
Reciprocal		Contains reciprocal edges
Directed cycles		Contains directed cycles
Loops		Does not contain loops

Statistics

Size	n =	2,239
Volume	m =	6,452
Loop count	l =	0
Wedge count	s =	353,779
Claw count	z =	21,373,531
Cross count	x =	1,277,691,904
Triangle count	t =	897
Square count	q =	166,681
4-Tour count	T₄ =	2,761,428
Maximum degree	d_max =	314
Maximum outdegree	d⁺_max =	314
Maximum indegree	d⁻_max =	19
Average degree	d =	5.763 29
Fill	p =	0.001 287 60
Size of LCC	N =	2,217
Size of LSCC	N_s =	8
Relative size of LSCC	N^r_s =	0.003 573 02
Diameter	δ =	10
50-Percentile effective diameter	δ_0.5 =	3.496 74
90-Percentile effective diameter	δ_0.9 =	4.833 42
Median distance	δ_M =	4
Mean distance	δ_m =	3.978 68
Gini coefficient	G =	0.671 702
Balanced inequality ratio	P =	0.236 671
Outdegree balanced inequality ratio	P₊ =	0.206 913
Indegree balanced inequality ratio	P₋ =	0.298 822
Relative edge distribution entropy	H_er =	0.854 883
Power law exponent	γ =	2.092 69
Tail power law exponent	γ_t =	1.721 00
Tail power law exponent with p	γ₃ =	1.721 00
p-value	p =	0.000 00
Outdegree tail power law exponent with p	γ_3,o =	1.691 00
Outdegree p-value	p_o =	0.001 000 00
Indegree tail power law exponent with p	γ_3,i =	1.851 00
Indegree p-value	p_i =	0.000 00
Degree assortativity	ρ =	−0.330 527
Degree assortativity p-value	p_ρ =	0.000 00
In/outdegree correlation	ρ^± =	−0.286 507
Clustering coefficient	c =	0.007 606 44
Directed clustering coefficient	c^± =	0.139 434
Spectral norm	α =	31.581 2
Operator 2-norm	ν =	30.777 6
Cyclic eigenvalue	π =	2.768 80
Algebraic connectivity	a =	0.102 530
Spectral separation	\|λ₁[A] / λ₂[A]\| =	1.050 19
Reciprocity	y =	0.006 199 63
Non-bipartivity	b_A =	0.047 786 8
Normalized non-bipartivity	b_N =	0.050 174 8
Algebraic non-bipartivity	χ =	0.104 135
Spectral bipartite frustration	b_K =	0.004 496 47
Controllability	C =	1,906
Relative controllability	C_r =	0.851 273

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

In/outdegree scatter plot

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

Matrix decompositions plots

Downloads

References

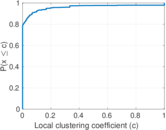
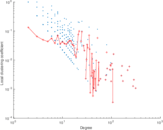
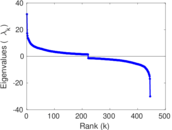
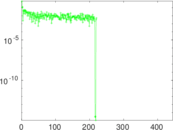
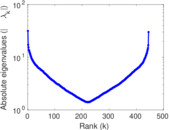
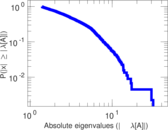
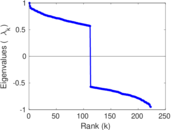
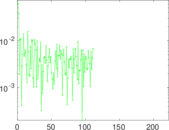
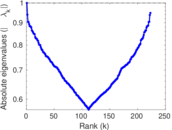
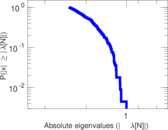
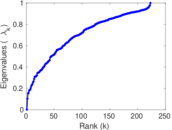
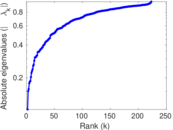
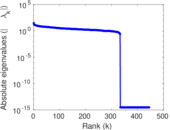
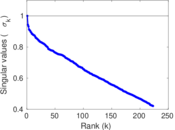
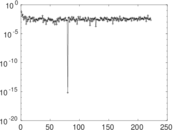
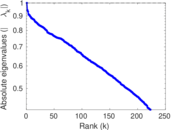
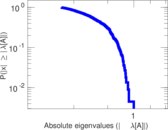
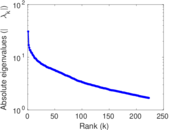
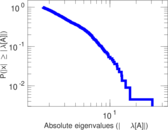
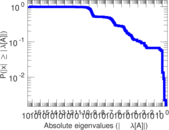
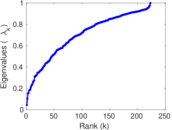
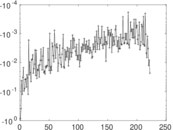
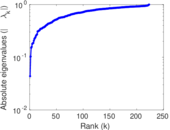
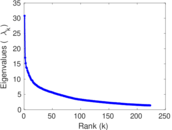
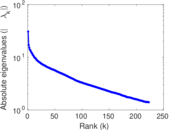
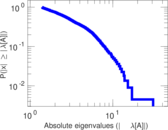
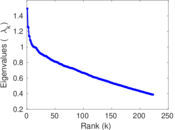
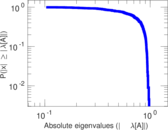
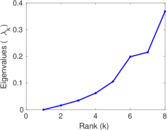
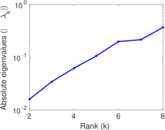
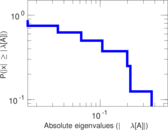
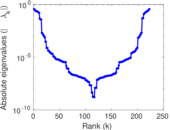
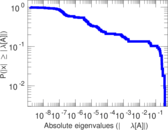
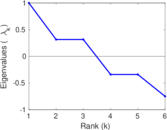
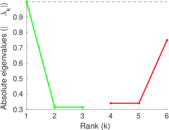
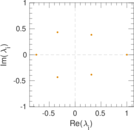
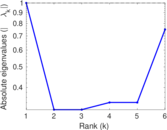
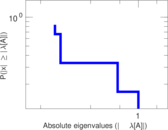
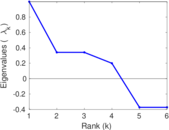
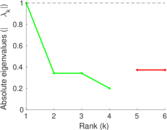
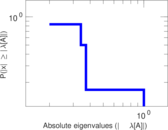
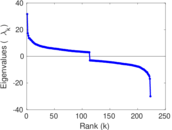
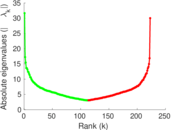
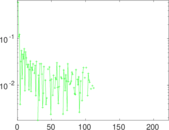
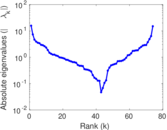
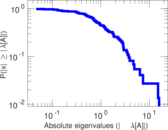
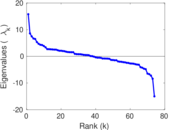
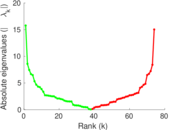
[1]

Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]

[2]

Rob M. Ewing, Peter Chu, Fred Elisma, Hongyan Li, Paul Taylor, Shane Climie, Linda McBroom-Cerajewski, Mark D. Robinson, Liam O'Connor, Michael Li, Rod Taylor, Moyez Dharsee, Yuen Ho, Adrian Heilbut, Lynda Moore, Shudong Zhang, Olga Ornatsky, Yury V. Bukhman, Martin Ethier, Yinglun Sheng, Julian Vasilescu, Mohamed Abu-Farha, Jean-Philippe P. Lambert, Henry S. Duewel, Ian I. Stewart, Bonnie Kuehl, Kelly Hogue, Karen Colwill, Katharine Gladwish, Brenda Muskat, Robert Kinach, Sally-Lin L. Adams, Michael F. Moran, Gregg B. Morin, Thodoros Topaloglou, and Daniel Figeys. Large-scale mapping of human protein–protein interactions by mass spectrometry. Molecular Systems Biol., 3, 2007.