PDZBase

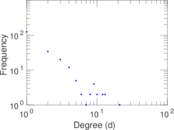
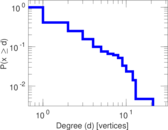
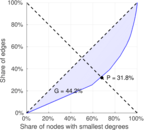
This is a network of protein–protein interactions from PDZBase.

Metadata

Code		`MP`
Internal name		`maayan-pdzbase`
Name		PDZBase
Data source		http://research.mssm.edu/maayan/datasets/qualitative_networks.shtml
Availability		Dataset is available for download
Consistency check		Dataset passed all tests
Category		Metabolic network
Node meaning		Protein
Edge meaning		Interaction
Network format		Unipartite, undirected
Edge type		Unweighted, no multiple edges
Loops		Contains loops

Size	n =	212
Volume	m =	244
Loop count	l =	2
Wedge count	s =	1,049
Claw count	z =	3,221
Cross count	x =	9,571
Triangle count	t =	1
Square count	q =	122
4-Tour count	T₄ =	5,656
Maximum degree	d_max =	21
Average degree	d =	2.301 89
Fill	p =	0.010 807 0
Size of LCC	N =	161
Diameter	δ =	10
50-Percentile effective diameter	δ_0.5 =	4.718 88
90-Percentile effective diameter	δ_0.9 =	7.206 09
Median distance	δ_M =	5
Mean distance	δ_m =	5.114 56
Gini coefficient	G =	0.442 178
Balanced inequality ratio	P =	0.317 623
Relative edge distribution entropy	H_er =	0.924 723
Power law exponent	γ =	3.006 86
Tail power law exponent	γ_t =	2.091 00
Tail power law exponent with p	γ₃ =	2.091 00
p-value	p =	0.009 000 00
Degree assortativity	ρ =	−0.356 716
Degree assortativity p-value	p_ρ =	5.712 88 × 10⁻¹⁶
Clustering coefficient	c =	0.002 859 87
Spectral norm	α =	5.840 66
Algebraic connectivity	a =	0.045 973 0
Spectral separation	\|λ₁[A] / λ₂[A]\| =	1.000 15
Non-bipartivity	b_A =	0.000 152 507
Normalized non-bipartivity	b_N =	0.002 569 68
Algebraic non-bipartivity	χ =	0.006 661 35
Spectral bipartite frustration	b_K =	0.000 641 433
Controllability	C =	100
Relative controllability	C_r =	0.471 698

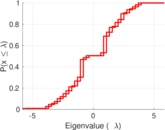
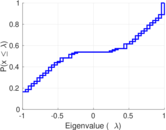
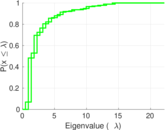
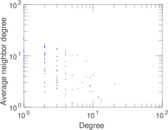
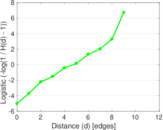
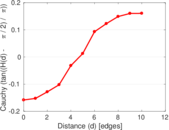
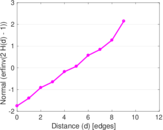
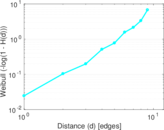
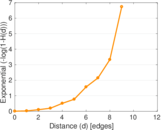
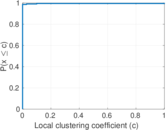
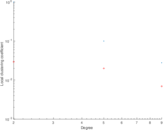
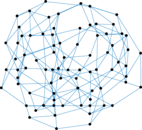
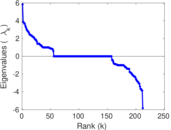
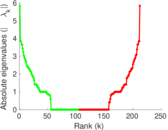
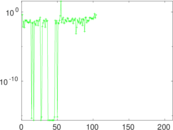
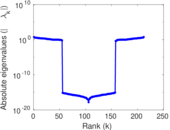
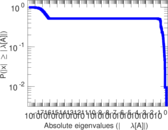
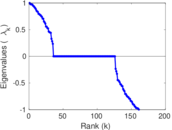
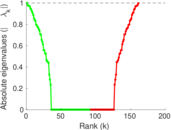
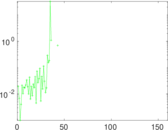
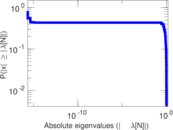
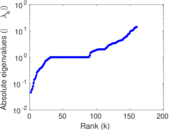
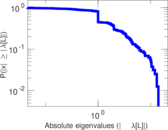
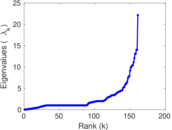
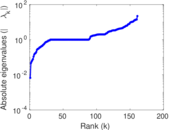
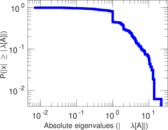
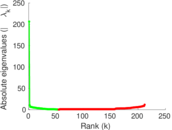
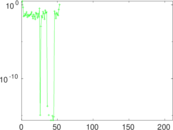
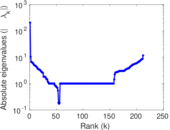
[1]	Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2]	Thijs Beuming, Lucy Skrabanek, Masha Y. Niv, Piali Mukherjee, and Harel Weinstein. PDZBase: A protein–protein interaction database for PDZ-domains. Bioinformatics, 21(6):827–828, 2005.