Windsurfers

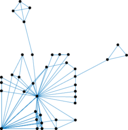
This undirected network contains interpersonal contacts between windsurfers in southern California during the fall of 1986. A node represents a windsurfer and an edge between two windsurfers shows that there was a interpersonal contact.

Metadata

CodeMW
Internal namemoreno_beach
NameWindsurfers
Data sourcehttp://moreno.ss.uci.edu/data.html#beach
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Human contact network
Node meaningPerson
Edge meaningContact
Network formatUnipartite, undirected
Edge typePositive weights, no multiple edges
LoopsDoes not contain loops

Statistics

Size n =43
Volume m =336
Loop count l =0
Wedge count s =5,831
Claw count z =36,530
Cross count x =180,422
Triangle count t =1,096
Square count q =12,034
4-Tour count T4 =120,268
Maximum degree dmax =31
Average degree d =15.627 9
Fill p =0.372 093
Size of LCC N =43
Diameter δ =3
50-Percentile effective diameter δ0.5 =1.265 55
90-Percentile effective diameter δ0.9 =1.926 56
Median distance δM =2
Mean distance δm =1.696 51
Gini coefficient G =0.237 403
Balanced inequality ratio P =0.409 226
Relative edge distribution entropy Her =0.976 716
Power law exponent γ =2.157 90
Tail power law exponent γt =5.021 00
Tail power law exponent with p γ3 =5.021 00
p-value p =0.286 000
Degree assortativity ρ =−0.146 965
Degree assortativity p-value pρ =0.000 131 592
Clustering coefficient c =0.563 883
Spectral norm α =125.530
Algebraic connectivity a =6.697 93
Spectral separation |λ1[A] / λ2[A]| =2.219 41
Non-bipartivity bA =0.724 591
Normalized non-bipartivity bN =0.678 918
Algebraic non-bipartivity χ =5.586 32
Spectral bipartite frustration bK =0.089 364 5
Controllability C =0
Relative controllability Cr =0.000 00

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

Edge weight/multiplicity distribution

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

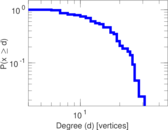
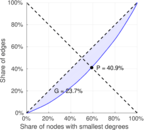
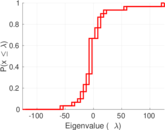
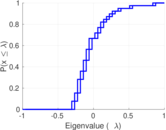
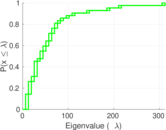
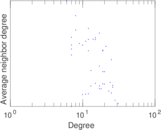
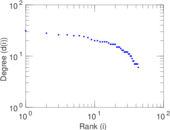
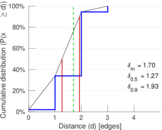
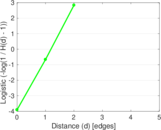
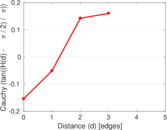
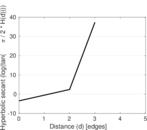
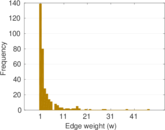
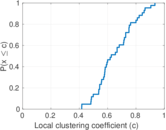
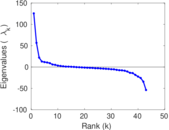
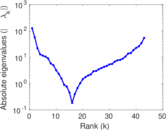
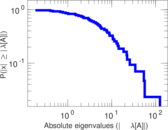
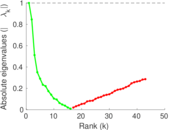
Matrix decompositions plots

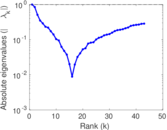
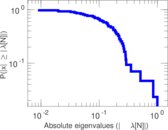
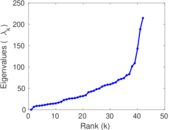
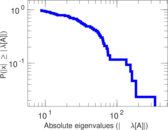
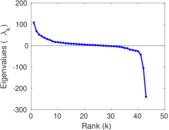
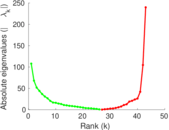
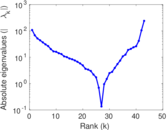
Downloads

References

[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] Linton Clarke Freeman, Cynthia Marie Webster, and Deirdre M. Kirke. Exploring social structure using dynamic three-dimensional color images. Soc. Netw., 20(2):109–118, 1998.
[3] Linton C. Freeman, Sue C. Freeman, and Alaina G. Michaelson. On human social intelligence. J. Soc. and Biol. Struct., 11(4):415–425, 1988.