Cattle

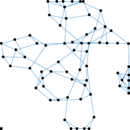
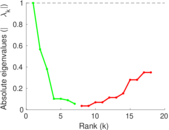
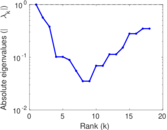
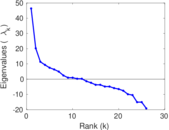
This directed network describes the dominance behavior observed between dairy cattle (Bos taurus) at the Iberia Livestock Experiment Station in Jenerette, Louisiana, USA. A node represents a cow and an edge represents the dominance of the left cow over the right cow. The edge weights indicate how often this besting behavior was observed.

Metadata

CodeMA
Internal namemoreno_cattle
NameCattle
Data sourcehttp://moreno.ss.uci.edu/data.html#cattle
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Animal network
Dataset timestamp 1955
Node meaningCattle
Edge meaningDominance
Network formatUnipartite, directed
Edge typePositive weights, no multiple edges
ReciprocalContains reciprocal edges
Directed cyclesContains directed cycles
LoopsDoes not contain loops
Skew-symmetry Inverted edges can be interpreted as negated edges

Statistics

Size n =28
Volume m =217
Loop count l =0
Wedge count s =3,136
Claw count z =19,026
Cross count x =75,584
Triangle count t =678
Square count q =7,216
4-Tour count T4 =70,682
Maximum degree dmax =24
Maximum outdegree d+max =18
Maximum indegree d−max =17
Average degree d =15.500 0
Fill p =0.287 037
Size of LCC N =28
Size of LSCC Ns =20
Relative size of LSCC Nrs =0.714 286
Diameter δ =3
50-Percentile effective diameter δ0.5 =0.959 584
90-Percentile effective diameter δ0.9 =1.832 84
Median distance δM =1
Mean distance δm =1.472 38
Gini coefficient G =0.181 698
Balanced inequality ratio P =0.412 442
Outdegree balanced inequality ratio P+ =0.341 014
Indegree balanced inequality ratio P− =0.377 880
Relative edge distribution entropy Her =0.978 603
Power law exponent γ =1.388 81
Tail power law exponent γt =5.411 00
Tail power law exponent with p γ3 =5.411 00
p-value p =0.097 000 0
Outdegree tail power law exponent with p γ3,o =5.221 00
Outdegree p-value po =0.241 000
Indegree tail power law exponent with p γ3,i =5.291 00
Indegree p-value pi =0.464 000
Degree assortativity ρ =−0.132 868
Degree assortativity p-value pρ =0.007 057 49
In/outdegree correlation ρ± =−0.249 901
Clustering coefficient c =0.648 597
Directed clustering coefficient c± =0.599 535
Spectral norm α =45.044 5
Operator 2-norm ν =29.657 9
Cyclic eigenvalue π =9.614 14
Algebraic connectivity a =1.988 20
Spectral separation |λ1[A] / λ2[A]| =2.240 31
Reciprocity y =0.110 599
Non-bipartivity bA =0.722 318
Normalized non-bipartivity bN =0.671 382
Algebraic non-bipartivity χ =0.941 479
Spectral bipartite frustration bK =0.016 074 0
Controllability C =3
Relative controllability Cr =0.107 143

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

In/outdegree scatter plot

Edge weight/multiplicity distribution

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

Matrix decompositions plots

Downloads

References

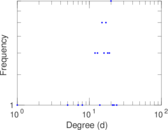
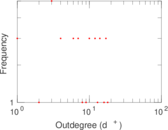
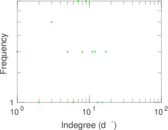
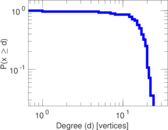
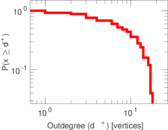
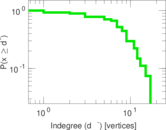
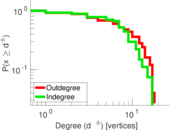
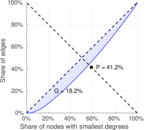
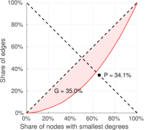
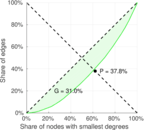
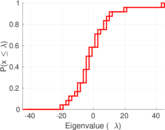
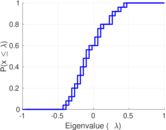
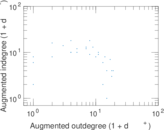
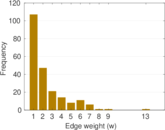
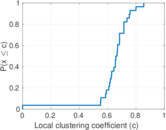
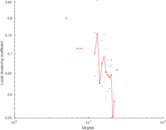
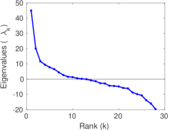
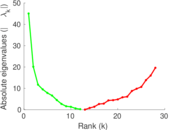
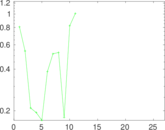
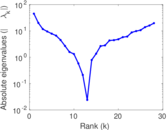
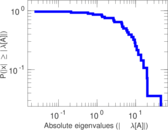
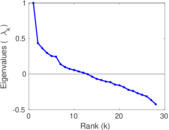
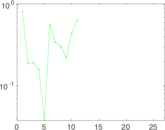
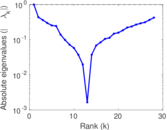
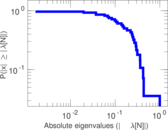
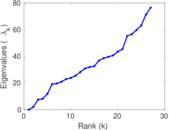
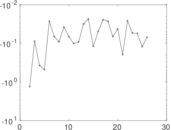
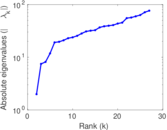
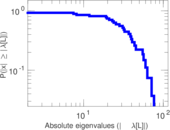
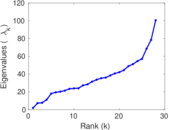
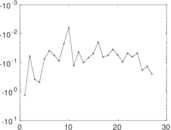
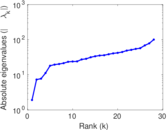
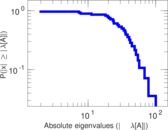
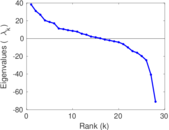
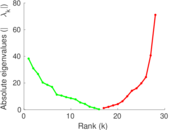
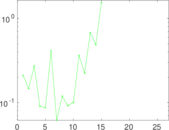
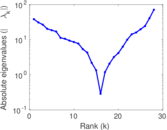
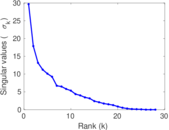
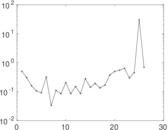
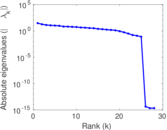
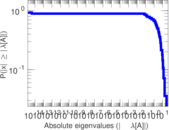
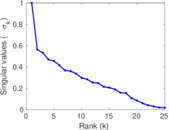
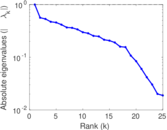
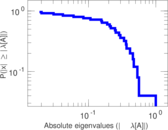
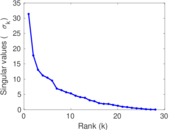
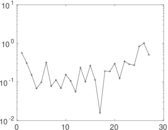
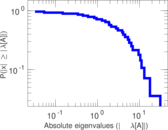
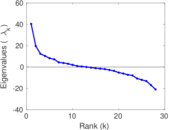
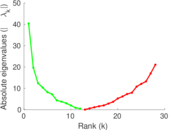
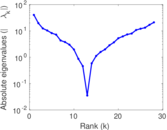
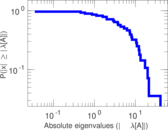
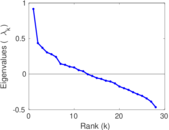
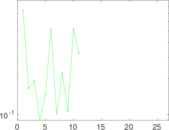
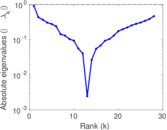
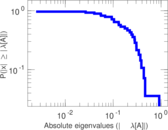
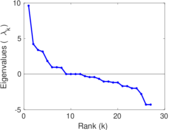
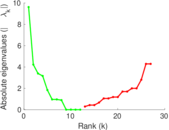
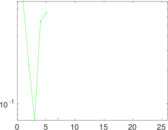
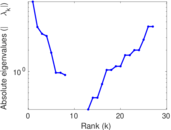
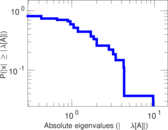
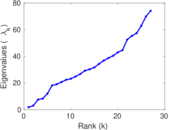
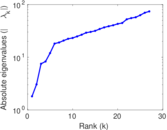
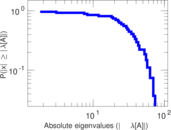
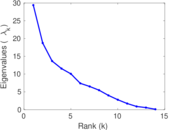
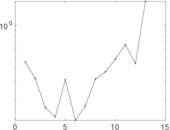
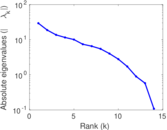
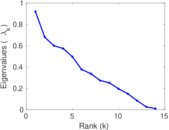
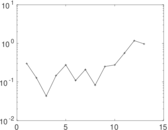
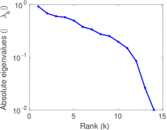
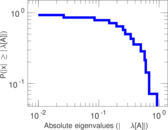
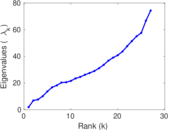
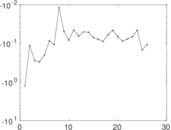
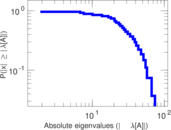
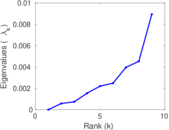
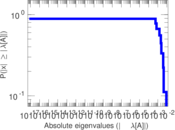
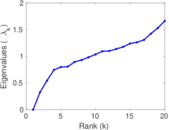
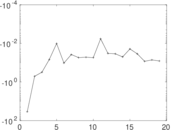
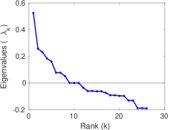
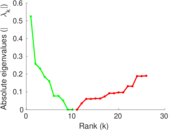
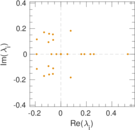
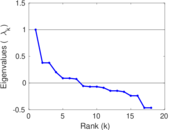
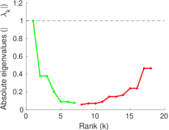
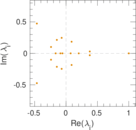
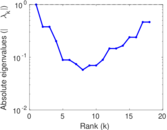
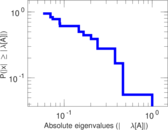
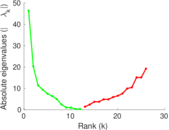
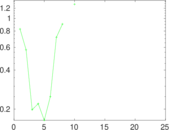
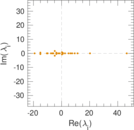
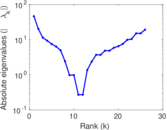
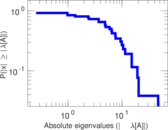
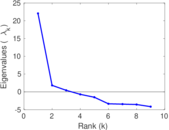
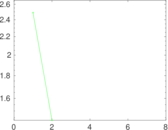
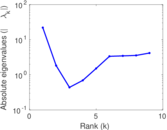
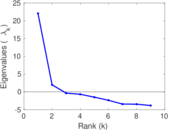
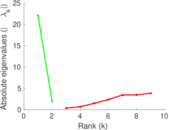
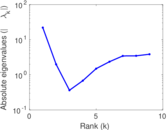
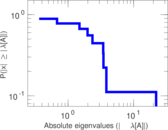
[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] Martin W. Schein and Milton H. Fohrman. Social dominance relationships in a herd of dairy cattle. The Br. J. of Anim. Behav., 3(2):45–55, 1955.