Sheep

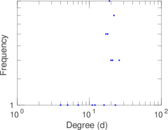
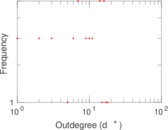
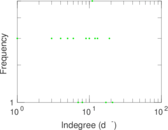
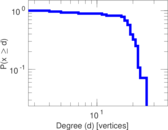
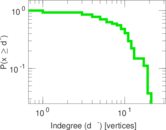
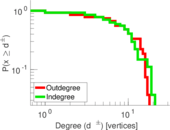
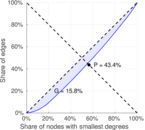
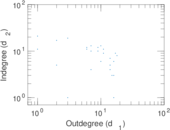
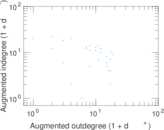
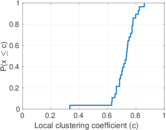
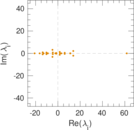
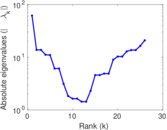
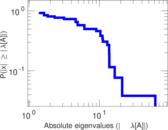
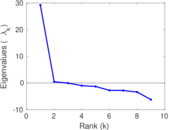
This directed network describes the dominance relationships between 28 female bighorn sheep (Ovis canadensis) observed on the National Bison Range in western Montana (USA) in 1984. A node represents a female bighorn sheep and an edge represents dominance of the left female bighorn sheep over the right female bighorn sheep. The edge weights indicate how often this dominance behavior was observed. Sheeps that are listed as nine years old may have been older in reality.

Metadata

CodeMc
Internal namemoreno_sheep
NameSheep
Data sourcehttp://moreno.ss.uci.edu/data.html#sheep
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Animal network
Dataset timestamp 1984
Node meaningSheep
Edge meaningDominance
Network formatUnipartite, directed
Edge typePositive weights, no multiple edges
ReciprocalContains reciprocal edges
Directed cyclesContains directed cycles
LoopsDoes not contain loops
Skew-symmetry Inverted edges can be interpreted as negated edges

Statistics

Size n =28
Volume m =250
Loop count l =0
Wedge count s =4,052
Claw count z =28,398
Cross count x =128,061
Triangle count t =983
Square count q =12,006
4-Tour count T4 =112,726
Maximum degree dmax =26
Maximum outdegree d+max =18
Maximum indegree d−max =21
Average degree d =17.857 1
Fill p =0.330 688
Size of LCC N =28
Size of LSCC Ns =22
Relative size of LSCC Nrs =0.785 714
Diameter δ =3
50-Percentile effective diameter δ0.5 =0.821 146
90-Percentile effective diameter δ0.9 =1.755 14
Median distance δM =1
Mean distance δm =1.369 79
Gini coefficient G =0.158 429
Balanced inequality ratio P =0.434 000
Outdegree balanced inequality ratio P+ =0.352 000
Indegree balanced inequality ratio P− =0.364 000
Relative edge distribution entropy Her =0.983 126
Power law exponent γ =1.733 13
Tail power law exponent γt =8.991 00
Tail power law exponent with p γ3 =8.991 00
p-value p =0.274 000
Outdegree tail power law exponent with p γ3,o =8.431 00
Outdegree p-value po =0.169 000
Indegree tail power law exponent with p γ3,i =3.981 00
Indegree p-value pi =0.464 000
Degree assortativity ρ =−0.004 000 26
Degree assortativity p-value pρ =0.931 075
In/outdegree correlation ρ± =−0.338 766
Clustering coefficient c =0.727 789
Directed clustering coefficient c± =0.643 463
Spectral norm α =59.891 9
Operator 2-norm ν =38.353 9
Cyclic eigenvalue π =13.811 6
Algebraic connectivity a =3.972 30
Spectral separation |λ1[A] / λ2[A]| =2.772 90
Reciprocity y =0.120 000
Non-bipartivity bA =0.755 225
Normalized non-bipartivity bN =0.664 061
Algebraic non-bipartivity χ =3.653 32
Spectral bipartite frustration bK =0.054 411 2
Controllability C =1
Relative controllability Cr =0.035 714 3

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

In/outdegree scatter plot

Edge weight/multiplicity distribution

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

Matrix decompositions plots

Downloads

References

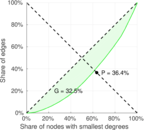
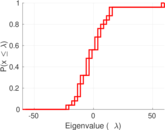
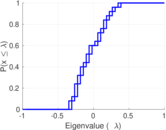
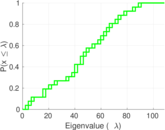
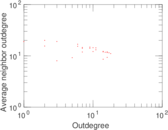
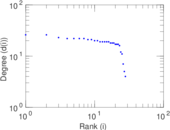
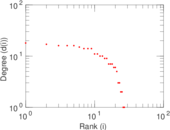
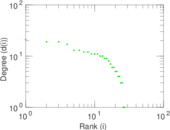
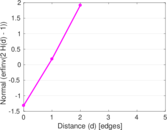
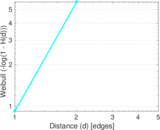
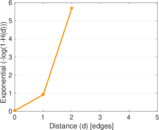
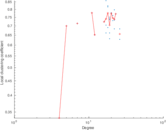
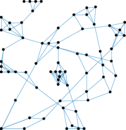
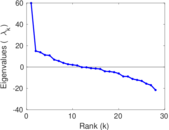
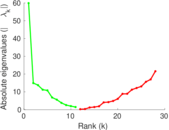
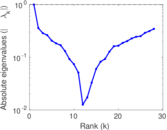
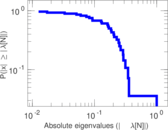
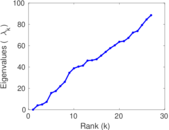
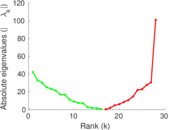
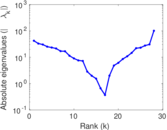
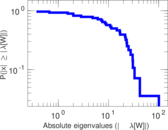
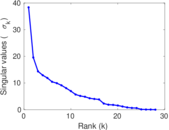
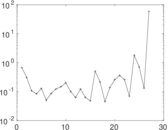
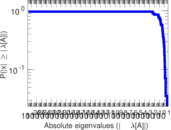
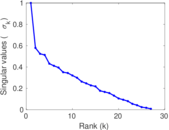
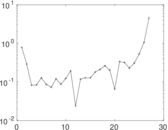
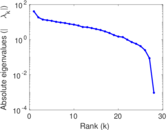
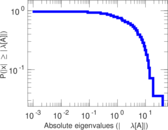
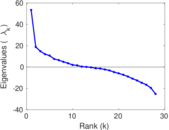
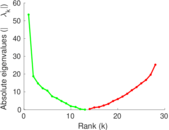
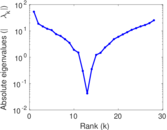
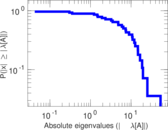
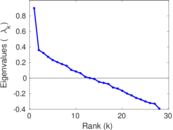
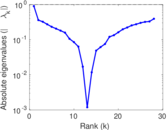
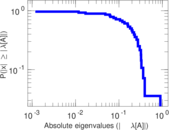
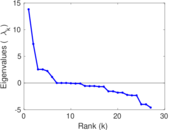
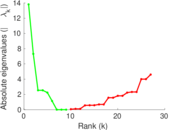
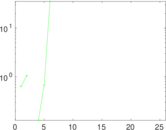
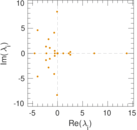
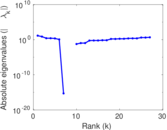
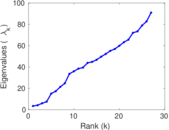
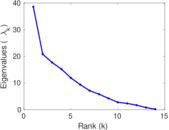
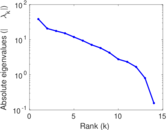
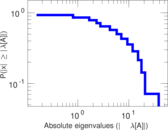
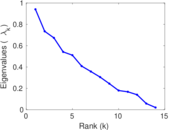
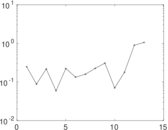
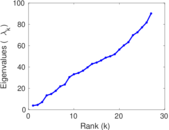
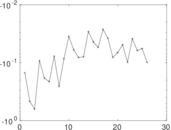
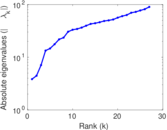
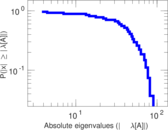
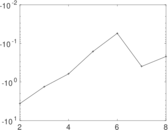
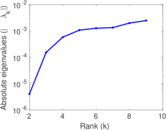
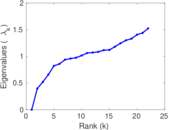
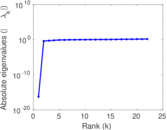
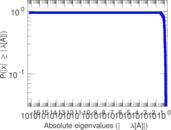
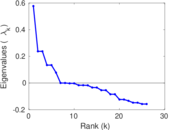
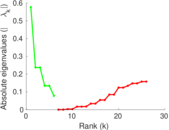
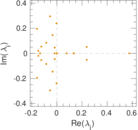
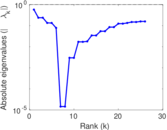
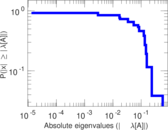
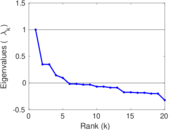
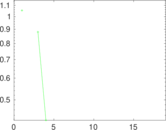
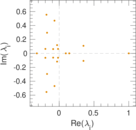
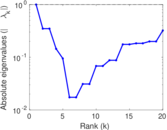
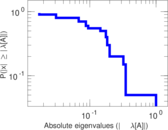
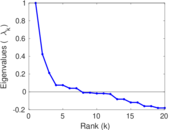
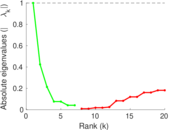
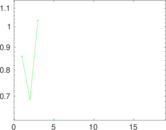
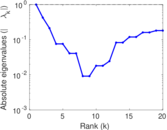
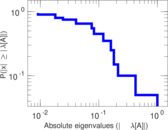
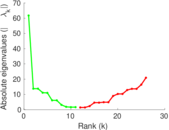
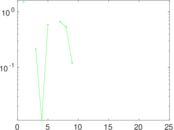
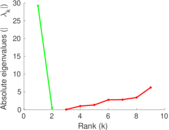
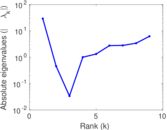
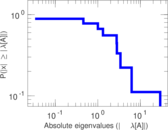
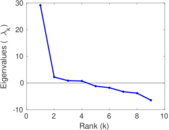
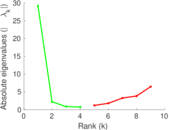
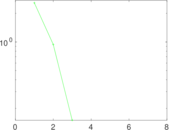
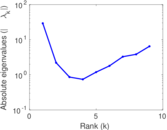
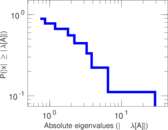
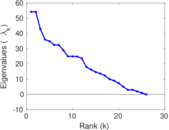
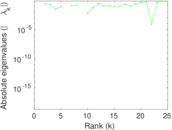
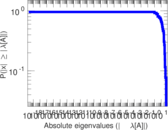
[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] Christine C. Hass. Social status in female bighorn sheep (Ovis canadensis): Expression, development and reproductive correlates. J. Zool., 225(3):509–523, 1991.