Taro exchange

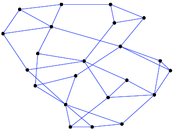
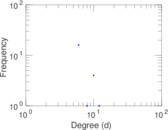
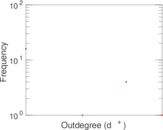
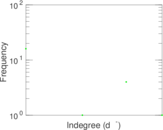
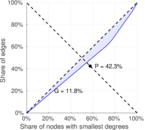
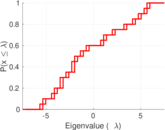
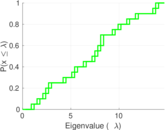
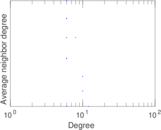
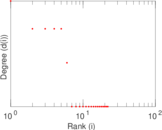
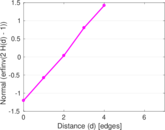
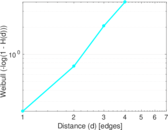
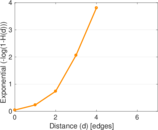
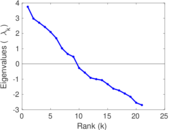
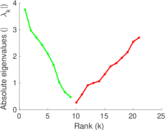
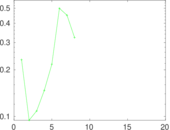
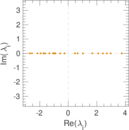
This undirected network contains gift-givings (taro) between households in a Papuan village. A node represents a household and an edge between two households indicates that there happened a gift-giving.

Metadata

Code		`MT`
Internal name		`moreno_taro`
Name		Taro exchange
Data source		http://moreno.ss.uci.edu/data.html#taro
Availability		Dataset is available for download
Consistency check		Dataset passed all tests
Category		Human social network
Node meaning		Household
Edge meaning		Gift-giving
Network format		Unipartite, directed
Edge type		Unweighted, no multiple edges
Reciprocal		Contains reciprocal edges
Directed cycles		Contains directed cycles
Loops		Does not contain loops

Statistics

Size	n =	22
Volume	m =	78
Loop count	l =	0
Wedge count	s =	109
Claw count	z =	1,076
Cross count	x =	1,645
Triangle count	t =	10
Square count	q =	4
4-Tour count	T₄ =	546
Maximum degree	d_max =	12
Maximum outdegree	d⁺_max =	6
Maximum indegree	d⁻_max =	6
Average degree	d =	7.090 91
Fill	p =	0.168 831
Size of LCC	N =	22
Size of LSCC	N_s =	22
Relative size of LSCC	N^r_s =	1.000 00
Diameter	δ =	5
50-Percentile effective diameter	δ_0.5 =	1.932 26
90-Percentile effective diameter	δ_0.9 =	3.259 62
Median distance	δ_M =	2
Mean distance	δ_m =	2.375 76
Gini coefficient	G =	0.117 716
Balanced inequality ratio	P =	0.423 077
Outdegree balanced inequality ratio	P₊ =	0.423 077
Indegree balanced inequality ratio	P₋ =	0.423 077
Relative edge distribution entropy	H_er =	0.989 648
Power law exponent	γ =	8.274 82
Tail power law exponent	γ_t =	8.991 00
Tail power law exponent with p	γ₃ =	8.991 00
p-value	p =	0.880 000
Outdegree tail power law exponent with p	γ_3,o =	8.991 00
Outdegree p-value	p_o =	0.851 000
Indegree tail power law exponent with p	γ_3,i =	8.991 00
Indegree p-value	p_i =	0.865 000
Degree assortativity	ρ =	−0.375 145
Degree assortativity p-value	p_ρ =	0.000 713 882
In/outdegree correlation	ρ^± =	+1.000 00
Clustering coefficient	c =	0.275 229
Directed clustering coefficient	c^± =	0.275 229
Spectral norm	α =	7.501 79
Operator 2-norm	ν =	3.750 90
Cyclic eigenvalue	π =	3.750 90
Algebraic connectivity	a =	1.072 52
Spectral separation	\|λ₁[A] / λ₂[A]\| =	1.261 79
Reciprocity	y =	1.000 00
Non-bipartivity	b_A =	0.280 755
Normalized non-bipartivity	b_N =	0.318 664
Algebraic non-bipartivity	χ =	1.051 84
Spectral bipartite frustration	b_K =	0.074 168 5
Controllability	C =	2
Relative controllability	C_r =	0.090 909 1

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

In/outdegree scatter plot

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

Matrix decompositions plots

Downloads

References

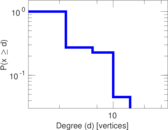
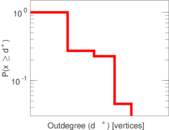
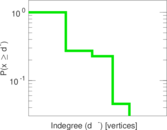
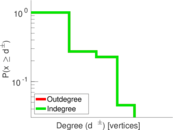
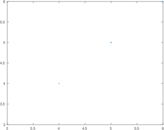
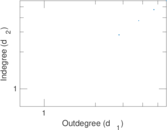
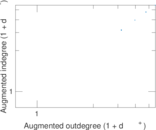
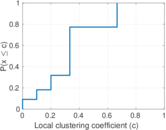
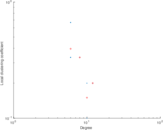
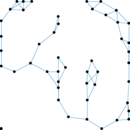
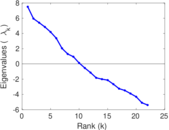
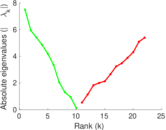
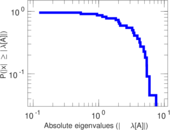
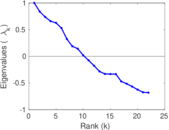
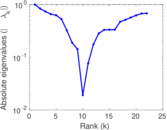
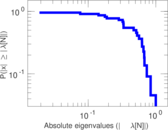
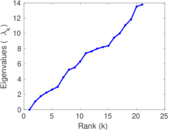
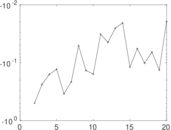
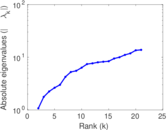
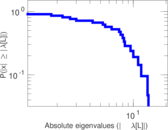
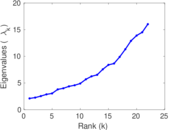
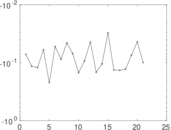
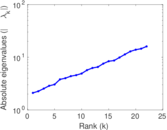
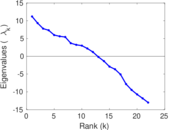
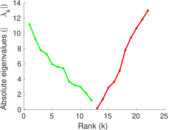
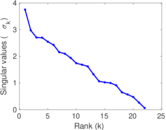
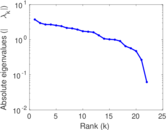
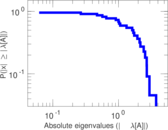
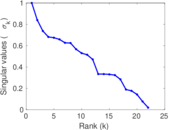
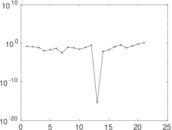
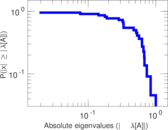
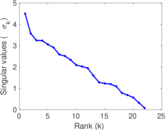
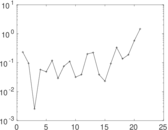
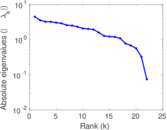
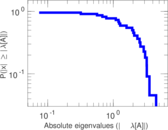
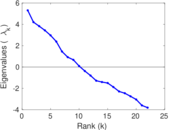
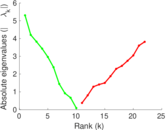
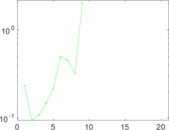
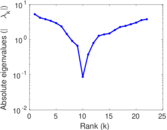
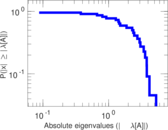
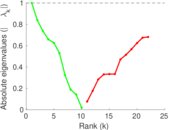
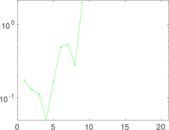
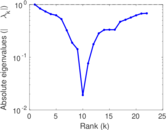
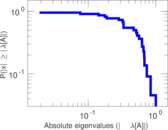
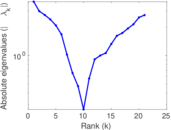
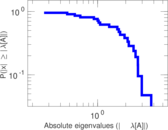
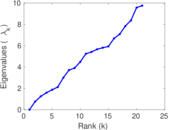
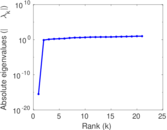
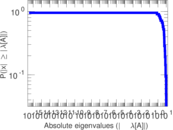
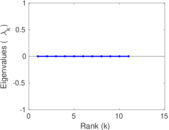
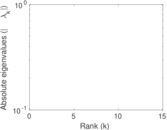
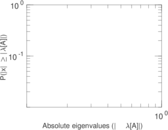
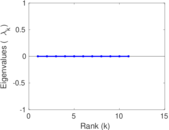
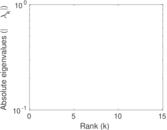
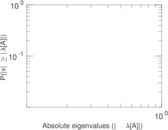
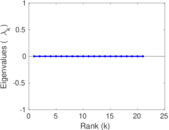
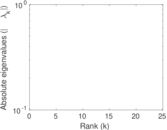
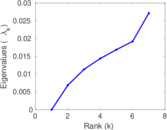
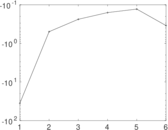
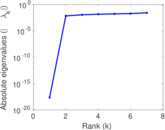
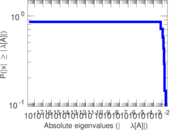
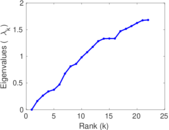
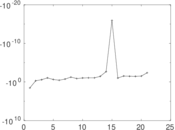
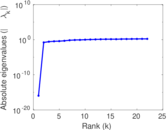
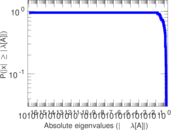
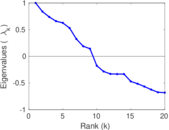
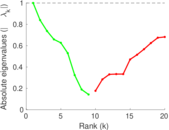
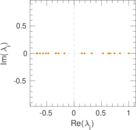
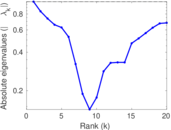
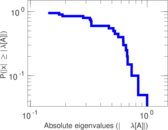
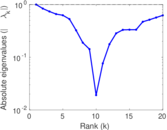
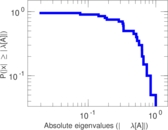
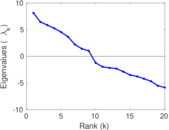
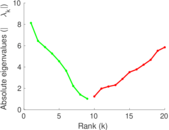
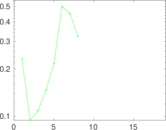
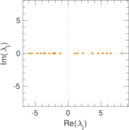
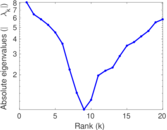
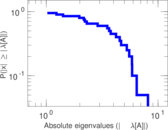
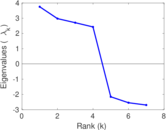
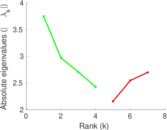
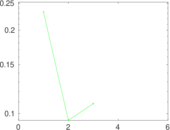
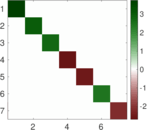
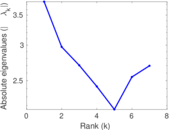
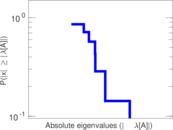
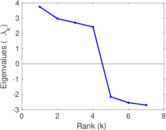
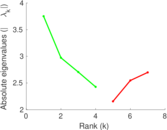
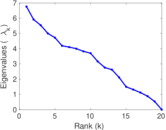
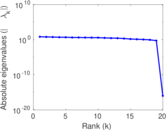
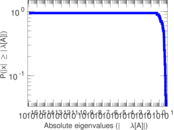
[1]	Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2]	Per Hage and Frank Harary. Structural Models in Anthropology. Cambridge Univ. Press, 1983.
[3]	Eric Schwimmer. Exchange in the Social Structure of the Orokaiva: Traditional and Emergent Ideologies in the Northern District of Papua. St. Martin's Press, 1973.