Gnutella (09)
This is a network of Gnutella hosts from 2002. The nodes represent Gnutella
hosts, and the directed edges represent connections between them. The dataset
is from August 09, 2002.
Metadata
Statistics
| Size | n = | 8,114
|
| Volume | m = | 26,013
|
| Loop count | l = | 0
|
| Wedge count | s = | 411,347
|
| Claw count | z = | 5,474,253
|
| Cross count | x = | 93,769,403
|
| Triangle count | t = | 2,354
|
| Square count | q = | 96,902
|
| 4-Tour count | T4 = | 2,472,630
|
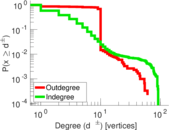
| Maximum degree | dmax = | 102
|
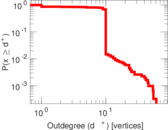
| Maximum outdegree | d+max = | 61
|
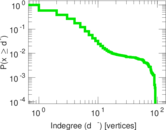
| Maximum indegree | d−max = | 92
|
| Average degree | d = | 6.411 88
|
| Fill | p = | 0.000 395 161
|
| Size of LCC | N = | 8,104
|
| Size of LSCC | Ns = | 2,624
|
| Relative size of LSCC | Nrs = | 0.323 392
|
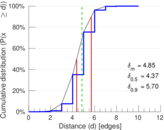
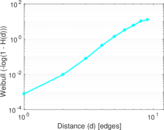
| Diameter | δ = | 10
|
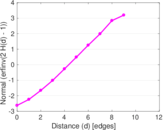
| 50-Percentile effective diameter | δ0.5 = | 4.367 96
|
| 90-Percentile effective diameter | δ0.9 = | 5.701 06
|
| Median distance | δM = | 5
|
| Mean distance | δm = | 4.847 71
|
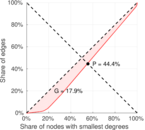
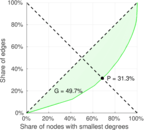
| Gini coefficient | G = | 0.529 167
|
| Balanced inequality ratio | P = | 0.298 851
|
| Outdegree balanced inequality ratio | P+ = | 0.443 624
|
| Indegree balanced inequality ratio | P− = | 0.313 382
|
| Relative edge distribution entropy | Her = | 0.942 194
|
| Power law exponent | γ = | 1.779 96
|
| Tail power law exponent | γt = | 4.731 00
|
| Tail power law exponent with p | γ3 = | 4.731 00
|
| p-value | p = | 0.000 00
|
| Outdegree tail power law exponent with p | γ3,o = | 2.591 00
|
| Outdegree p-value | po = | 0.134 000
|
| Indegree tail power law exponent with p | γ3,i = | 2.901 00
|
| Indegree p-value | pi = | 0.000 00
|
| Degree assortativity | ρ = | +0.033 224 1
|
| Degree assortativity p-value | pρ = | 3.453 89 × 10−14
|
| In/outdegree correlation | ρ± = | +0.167 968
|
| Clustering coefficient | c = | 0.017 168 0
|
| Directed clustering coefficient | c± = | 0.021 210 0
|
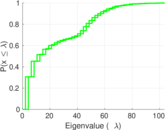
| Spectral norm | α = | 28.451 4
|
| Operator 2-norm | ν = | 24.865 4
|
| Cyclic eigenvalue | π = | 4.536 06
|
| Algebraic connectivity | a = | 0.068 316 9
|
| Spectral separation | |λ1[A] / λ2[A]| = | 1.290 55
|
| Reciprocity | y = | 0.000 00
|
| Non-bipartivity | bA = | 0.225 136
|
| Normalized non-bipartivity | bN = | 0.035 143 9
|
| Algebraic non-bipartivity | χ = | 0.068 085 9
|
| Spectral bipartite frustration | bK = | 0.002 651 92
|
| Controllability | C = | 5,357
|
| Relative controllability | Cr = | 0.660 217
|
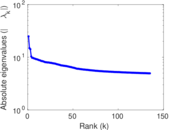
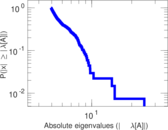
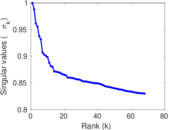
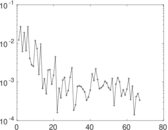
Plots

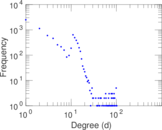
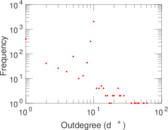
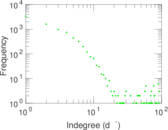
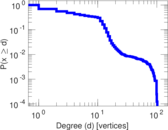



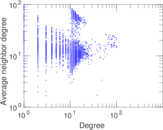

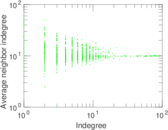


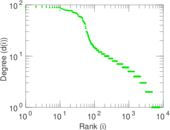



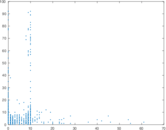
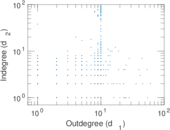
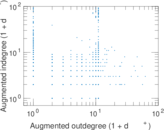

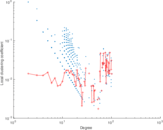
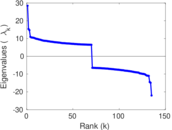
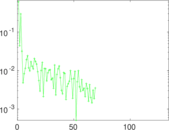
Matrix decompositions plots


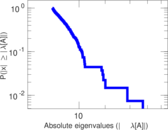
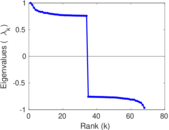
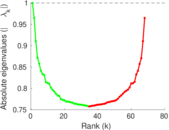
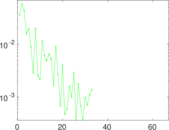



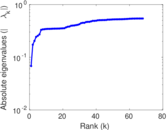
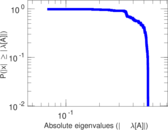

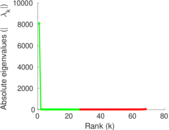






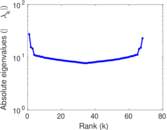
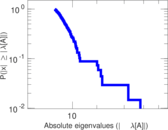
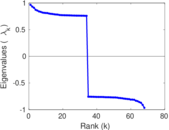

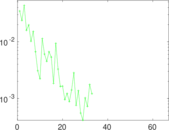
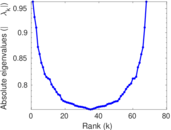
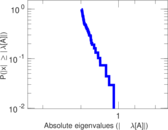
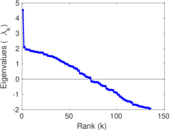
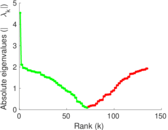
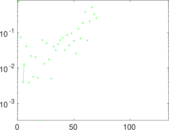

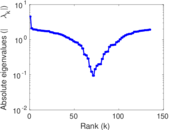
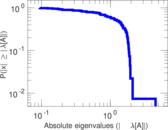
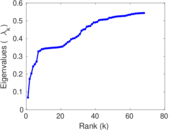


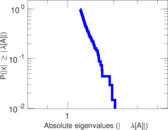
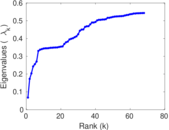
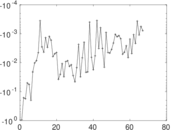
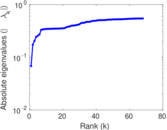
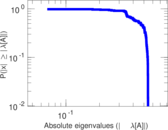
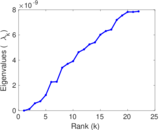
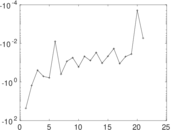
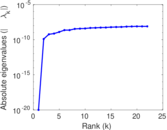
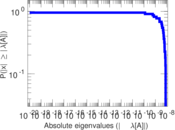
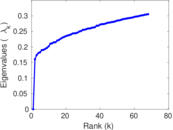

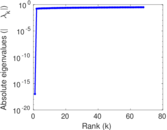

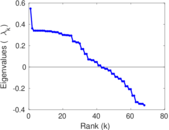
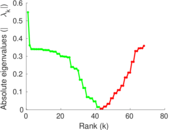
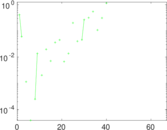

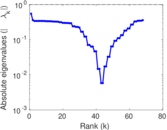
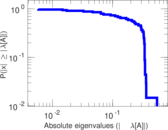

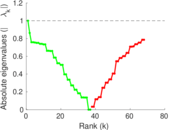

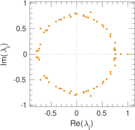
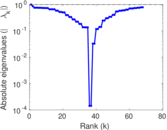


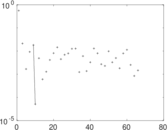
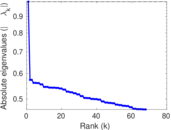
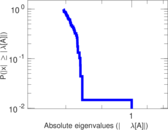

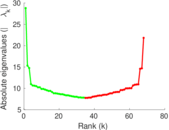
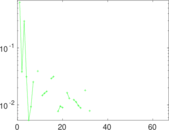
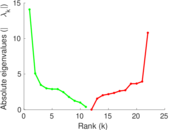
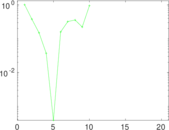
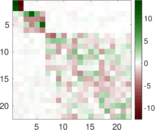
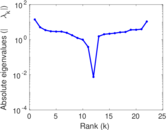
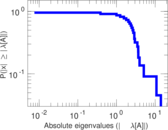

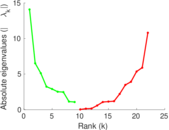
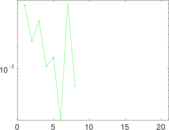
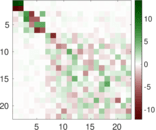
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Matei Ripeanu, Ian Foster, and Adriana Iamnitchi.
Mapping the Gnutella network: Properties of large-scale
peer-to-peer systems and implications for system design.
IEEE Internet Comput. J., 6, 2002.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee





















































































































































