Gnutella (24)
This is a network of Gnutella hosts from 2002. The nodes represent Gnutella
hosts, and the directed edges represent connections between them. The dataset
is from August 24, 2002.
Metadata
Statistics
| Size | n = | 26,518
|
| Volume | m = | 65,369
|
| Loop count | l = | 0
|
| Wedge count | s = | 721,206
|
| Claw count | z = | 10,489,472
|
| Cross count | x = | 665,822,688
|
| Triangle count | t = | 986
|
| Square count | q = | 8,794
|
| 4-Tour count | T4 = | 3,085,914
|
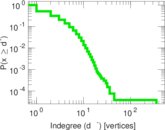
| Maximum degree | dmax = | 355
|
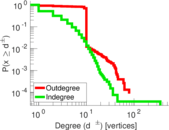
| Maximum outdegree | d+max = | 79
|
| Maximum indegree | d−max = | 355
|
| Average degree | d = | 4.930 16
|
| Fill | p = | 9.296 23 × 10−5
|
| Size of LCC | N = | 26,498
|
| Size of LSCC | Ns = | 6,352
|
| Relative size of LSCC | Nrs = | 0.239 535
|
| Diameter | δ = | 11
|
| 50-Percentile effective diameter | δ0.5 = | 5.094 74
|
| 90-Percentile effective diameter | δ0.9 = | 6.320 12
|
| Median distance | δM = | 6
|
| Mean distance | δm = | 5.531 11
|
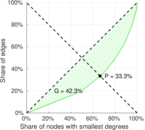
| Gini coefficient | G = | 0.546 890
|
| Balanced inequality ratio | P = | 0.272 759
|
| Outdegree balanced inequality ratio | P+ = | 0.449 326
|
| Indegree balanced inequality ratio | P− = | 0.333 384
|
| Relative edge distribution entropy | Her = | 0.948 052
|
| Power law exponent | γ = | 1.985 92
|
| Tail power law exponent | γt = | 6.271 00
|
| Tail power law exponent with p | γ3 = | 6.271 00
|
| p-value | p = | 0.000 00
|
| Outdegree tail power law exponent with p | γ3,o = | 2.571 00
|
| Outdegree p-value | po = | 0.052 000 0
|
| Indegree tail power law exponent with p | γ3,i = | 5.121 00
|
| Indegree p-value | pi = | 0.149 000
|
| Degree assortativity | ρ = | −0.007 728 27
|
| Degree assortativity p-value | pρ = | 0.005 199 95
|
| In/outdegree correlation | ρ± = | +0.292 007
|
| Clustering coefficient | c = | 0.004 101 46
|
| Directed clustering coefficient | c± = | 0.004 049 56
|
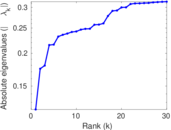
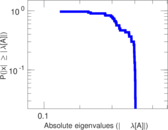
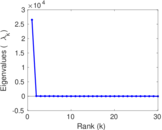
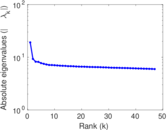
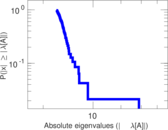
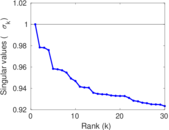
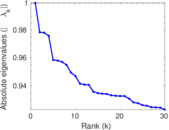
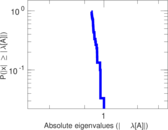
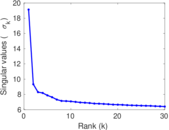
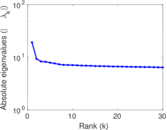
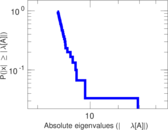
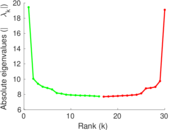
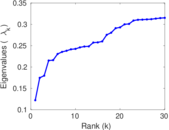
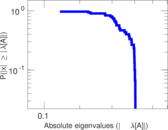
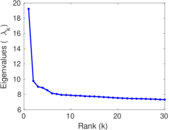
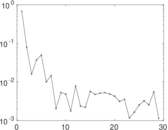
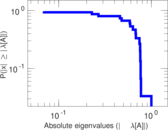
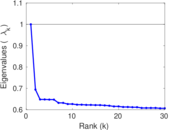
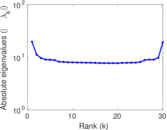
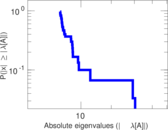
| Spectral norm | α = | 19.585 1
|
| Operator 2-norm | ν = | 19.116 0
|
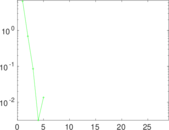
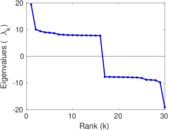
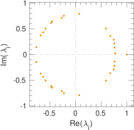
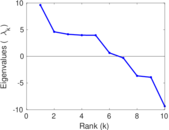
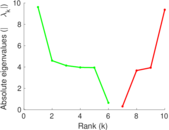
| Cyclic eigenvalue | π = | 3.545 78
|
| Algebraic connectivity | a = | 0.122 096
|
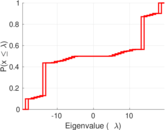
| Spectral separation | |λ1[A] / λ2[A]| = | 1.027 03
|
| Reciprocity | y = | 0.000 00
|
| Non-bipartivity | bA = | 0.026 316 2
|
| Normalized non-bipartivity | bN = | 0.065 985 4
|
| Algebraic non-bipartivity | χ = | 0.122 041
|
| Spectral bipartite frustration | bK = | 0.006 184 77
|
| Controllability | C = | 18,965
|
| Relative controllability | Cr = | 0.715 175
|
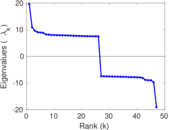
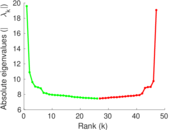
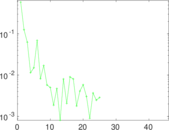
Plots

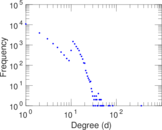

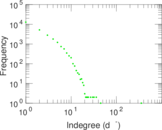
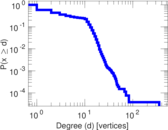
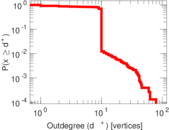



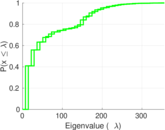



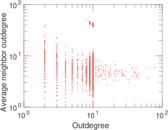
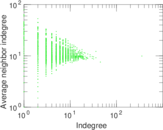
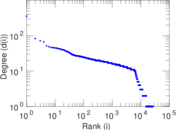
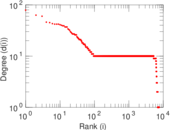
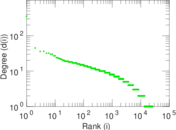
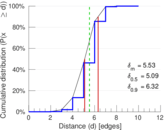
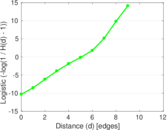
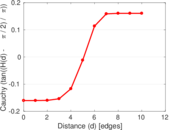
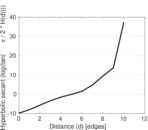
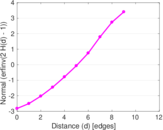
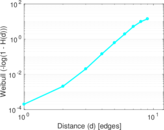







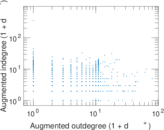

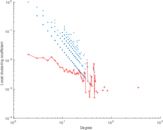
Matrix decompositions plots
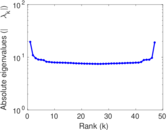
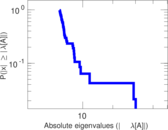
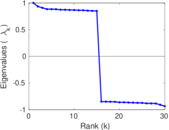
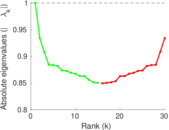

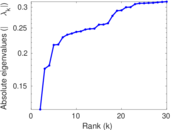
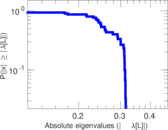
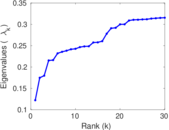
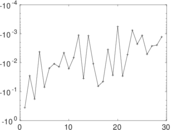

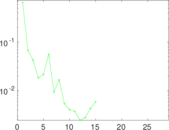
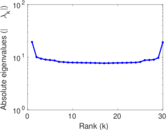
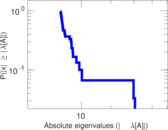

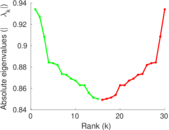
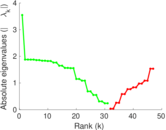

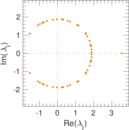
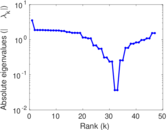
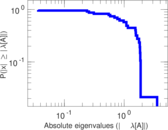




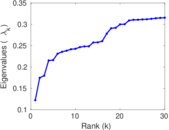

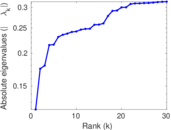
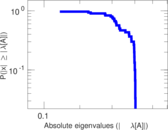

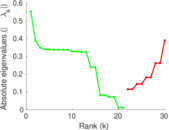

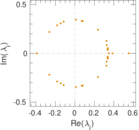
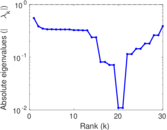
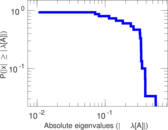




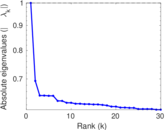

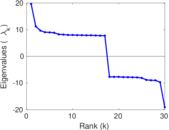

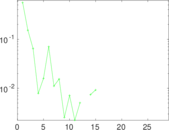
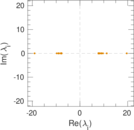
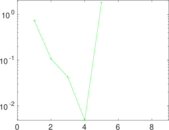

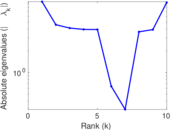
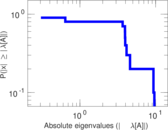

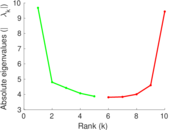


Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Matei Ripeanu, Ian Foster, and Adriana Iamnitchi.
Mapping the Gnutella network: Properties of large-scale
peer-to-peer systems and implications for system design.
IEEE Internet Comput. J., 6, 2002.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee






















































































































































