Gnutella (31)
This is a network of Gnutella hosts from 2002. The nodes represent Gnutella
hosts, and the directed edges represent connections between them. The dataset
is from August 31, 2002.
Metadata
Statistics
| Size | n = | 62,586
|
| Volume | m = | 147,892
|
| Loop count | l = | 0
|
| Wedge count | s = | 1,568,174
|
| Claw count | z = | 8,171,989
|
| Cross count | x = | 43,841,182
|
| Triangle count | t = | 2,024
|
| Square count | q = | 42,466
|
| 4-Tour count | T4 = | 6,908,208
|
| Maximum degree | dmax = | 95
|
| Maximum outdegree | d+max = | 78
|
| Maximum indegree | d−max = | 68
|
| Average degree | d = | 4.726 04
|
| Fill | p = | 3.775 70 × 10−5
|
| Size of LCC | N = | 62,561
|
| Size of LSCC | Ns = | 14,149
|
| Relative size of LSCC | Nrs = | 0.226 073
|
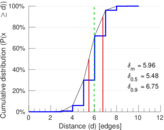
| Diameter | δ = | 11
|
| 50-Percentile effective diameter | δ0.5 = | 5.479 36
|
| 90-Percentile effective diameter | δ0.9 = | 6.751 31
|
| Median distance | δM = | 6
|
| Mean distance | δm = | 5.956 53
|
| Gini coefficient | G = | 0.562 578
|
| Balanced inequality ratio | P = | 0.263 936
|
| Outdegree balanced inequality ratio | P+ = | 0.455 637
|
| Indegree balanced inequality ratio | P− = | 0.330 897
|
| Relative edge distribution entropy | Her = | 0.948 442
|
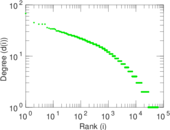
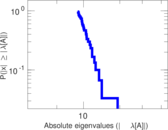
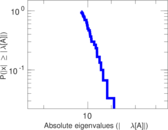
| Power law exponent | γ = | 2.062 94
|
| Tail power law exponent | γt = | 4.831 00
|
| Tail power law exponent with p | γ3 = | 4.831 00
|
| p-value | p = | 0.000 00
|
| Outdegree tail power law exponent with p | γ3,o = | 6.051 00
|
| Outdegree p-value | po = | 0.713 000
|
| Indegree tail power law exponent with p | γ3,i = | 5.561 00
|
| Indegree p-value | pi = | 0.210 000
|
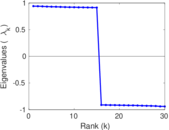
| Degree assortativity | ρ = | −0.092 557 8
|
| Degree assortativity p-value | pρ = | 0.000 00
|
| In/outdegree correlation | ρ± = | +0.288 763
|
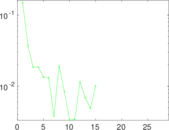
| Clustering coefficient | c = | 0.003 872 02
|
| Directed clustering coefficient | c± = | 0.003 653 97
|
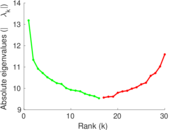
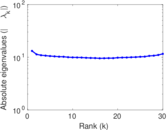
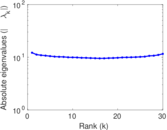
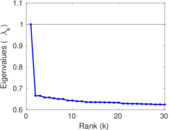
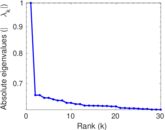
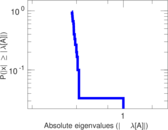
| Spectral norm | α = | 13.181 2
|
| Operator 2-norm | ν = | 10.896 2
|
| Cyclic eigenvalue | π = | 3.659 28
|
| Algebraic connectivity | a = | 0.113 127
|
| Spectral separation | |λ1[A] / λ2[A]| = | 1.137 32
|
| Reciprocity | y = | 0.000 00
|
| Non-bipartivity | bA = | 0.120 738
|
| Normalized non-bipartivity | bN = | 0.059 887 0
|
| Algebraic non-bipartivity | χ = | 0.113 103
|
| Spectral bipartite frustration | bK = | 0.005 981 14
|
| Controllability | C = | 46,227
|
| Relative controllability | Cr = | 0.738 616
|
Plots

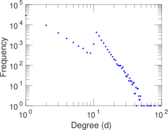
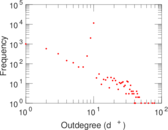

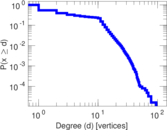




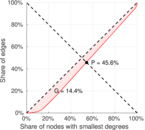


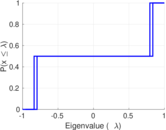
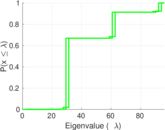


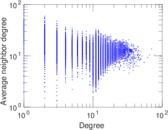
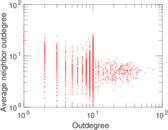
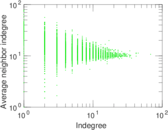
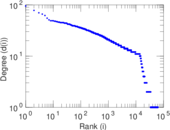
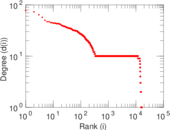





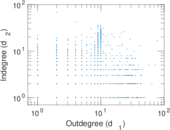

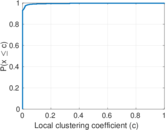
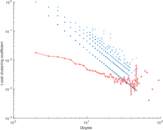

Matrix decompositions plots

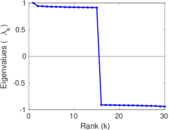
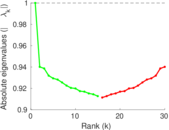
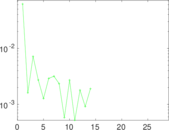


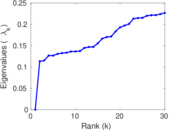
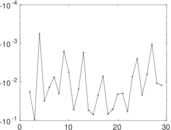

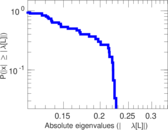
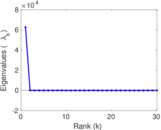
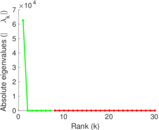
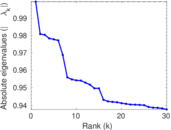
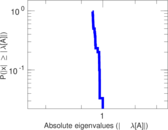
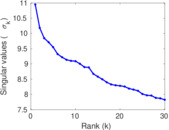

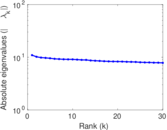

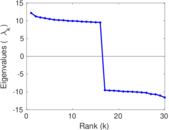
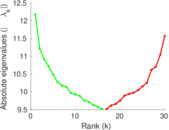
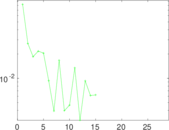

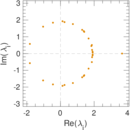
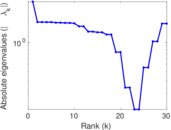
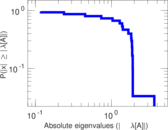
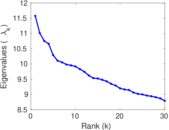
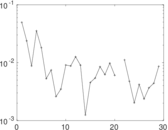
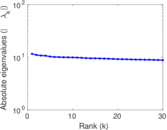
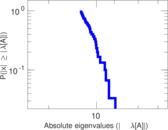
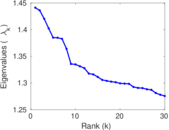


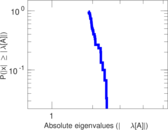


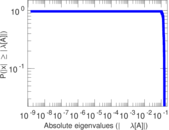

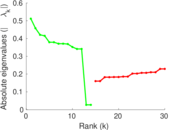
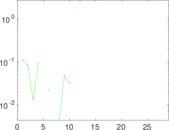
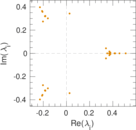
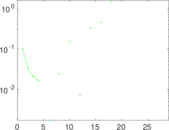
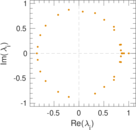
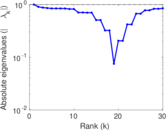
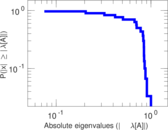
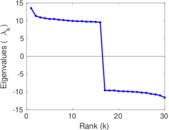
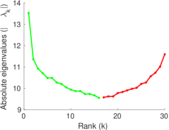
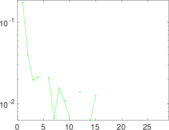
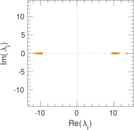
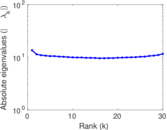

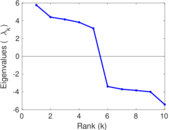
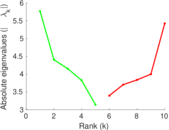

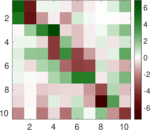

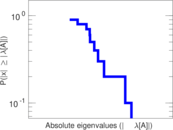
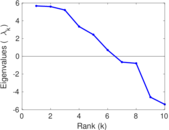


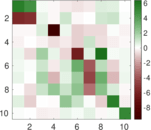
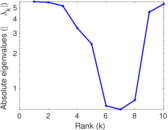
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Matei Ripeanu, Ian Foster, and Adriana Iamnitchi.
Mapping the Gnutella network: Properties of large-scale
peer-to-peer systems and implications for system design.
IEEE Internet Comput. J., 6, 2002.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee










































































































































