Hamsterster full
This Network contains friendships and familylinks between users of the website
hamsterster.com.
Metadata
Statistics
| Size | n = | 2,426
|
| Volume | m = | 16,631
|
| Loop count | l = | 0
|
| Wedge count | s = | 690,463
|
| Claw count | z = | 19,926,742
|
| Cross count | x = | 676,944,899
|
| Triangle count | t = | 53,265
|
| Square count | q = | 1,234,177
|
| 4-Tour count | T4 = | 12,668,530
|
| Maximum degree | dmax = | 273
|
| Average degree | d = | 13.710 6
|
| Fill | p = | 0.005 653 87
|
| Size of LCC | N = | 2,000
|
| Diameter | δ = | 10
|
| 50-Percentile effective diameter | δ0.5 = | 3.013 27
|
| 90-Percentile effective diameter | δ0.9 = | 4.797 16
|
| Median distance | δM = | 4
|
| Mean distance | δm = | 3.668 46
|
| Gini coefficient | G = | 0.589 364
|
| Balanced inequality ratio | P = | 0.276 291
|
| Relative edge distribution entropy | Her = | 0.917 954
|
| Power law exponent | γ = | 1.518 29
|
| Tail power law exponent | γt = | 2.421 00
|
| Tail power law exponent with p | γ3 = | 2.421 00
|
| p-value | p = | 0.000 00
|
| Degree assortativity | ρ = | +0.047 403 9
|
| Degree assortativity p-value | pρ = | 5.149 83 × 10−18
|
| Clustering coefficient | c = | 0.231 432
|
| Spectral norm | α = | 50.021 5
|
| Algebraic connectivity | a = | 0.102 938
|
| Spectral separation | |λ1[A] / λ2[A]| = | 1.608 94
|
| Non-bipartivity | bA = | 0.602 303
|
| Normalized non-bipartivity | bN = | 0.129 659
|
| Algebraic non-bipartivity | χ = | 0.193 235
|
| Spectral bipartite frustration | bK = | 0.003 000 91
|
| Controllability | C = | 63
|
| Relative controllability | Cr = | 0.025 968 7
|
Plots
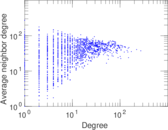
Matrix decompositions plots
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
KONECT ‣ Networks ‣
Buy Me a Coffee
