Reuters

This is the bipartite network of story–word inclusions in documents that appeared in Reuters news stories collected in the Reuters Corpus, Volume 1 (RCV1). Left nodes represent stories; right nodes represent words. An edge represents a story–word inclusion.

Metadata

CodeRE
Internal namereuters
NameReuters
Data sourcehttp://trec.nist.gov/data/reuters/reuters.html
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Text network
Node meaningStory, word
Edge meaningInclusion
Network formatBipartite, undirected
Edge typeUnweighted, multiple edges

Statistics

Size n =1,065,176
Left size n1 =781,265
Right size n2 =283,911
Volume m =96,903,520
Unique edge count m̿ =60,569,726
Wedge count s =1,546,388,153,215
Claw count z =64,992,574,078,209,184
Cross count x =2.950 23 × 1021
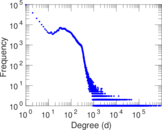
Maximum degree dmax =345,056
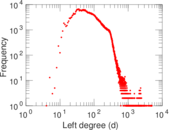
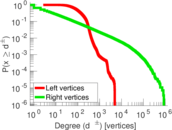
Maximum left degree d1max =1,585
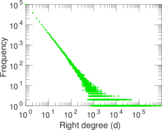
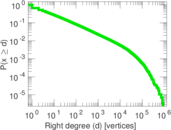
Maximum right degree d2max =345,056
Average degree d =181.948
Average left degree d1 =124.034
Average right degree d2 =341.317
Fill p =0.000 273 071
Average edge multiplicity m̃ =1.599 87
Size of LCC N =1,065,175
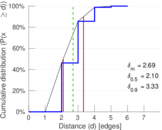
Diameter δ =6
50-Percentile effective diameter δ0.5 =2.097 31
90-Percentile effective diameter δ0.9 =3.331 29
Median distance δM =3
Mean distance δm =2.693 82
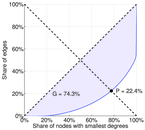
Gini coefficient G =0.680 191
Balanced inequality ratio P =0.247 840
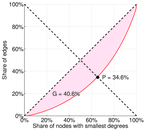
Left balanced inequality ratio P1 =0.345 764
Right balanced inequality ratio P2 =0.042 455 4
Relative edge distribution entropy Her =0.826 289
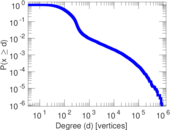
Power law exponent γ =1.295 75
Tail power law exponent γt =2.511 00
Degree assortativity ρ =−0.124 689
Degree assortativity p-value pρ =0.000 00
Spectral norm α =6,502.10
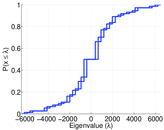
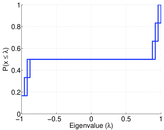
Spectral separation |λ1[A] / λ2[A]| =1.350 62

Plots

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the normalized adjacency matrix

Hop distribution

Edge weight/multiplicity distribution

Downloads

References

[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] David D. Lewis, Yiming Yang, Tony G. Rose, and Fan Li. RCV1: A new benchmark collection for text categorization research. J. Mach. Learn. Res., 5:361–397, 2004.