Epinions
This is the trust network from the online social network Epinions. Nodes are
users of Epinions and directed edges represent trust between the users.
Metadata
Statistics
| Size | n = | 75,879
|
| Volume | m = | 508,837
|
| Loop count | l = | 0
|
| Wedge count | s = | 74,201,120
|
| Claw count | z = | 27,111,469,575
|
| Cross count | x = | 9,737,182,087,729
|
| Triangle count | t = | 1,624,481
|
| Square count | q = | 166,635,817
|
| 4-Tour count | T4 = | 1,630,702,496
|
| Maximum degree | dmax = | 3,079
|
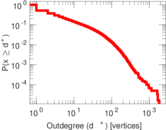
| Maximum outdegree | d+max = | 1,801
|
| Maximum indegree | d−max = | 3,035
|
| Average degree | d = | 13.411 8
|
| Fill | p = | 8.837 74 × 10−5
|
| Size of LCC | N = | 75,877
|
| Size of LSCC | Ns = | 32,223
|
| Relative size of LSCC | Nrs = | 0.424 663
|
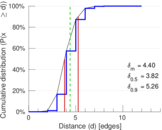
| Diameter | δ = | 15
|
| 50-Percentile effective diameter | δ0.5 = | 3.816 09
|
| 90-Percentile effective diameter | δ0.9 = | 5.258 77
|
| Median distance | δM = | 4
|
| Mean distance | δm = | 4.400 33
|
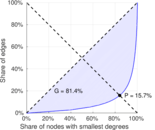
| Gini coefficient | G = | 0.814 342
|
| Balanced inequality ratio | P = | 0.157 292
|
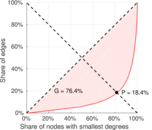
| Outdegree balanced inequality ratio | P+ = | 0.184 344
|
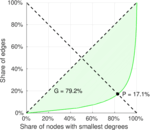
| Indegree balanced inequality ratio | P− = | 0.170 510
|
| Relative edge distribution entropy | Her = | 0.845 432
|
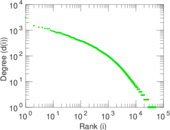
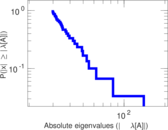
| Power law exponent | γ = | 2.025 80
|
| Tail power law exponent | γt = | 1.691 00
|
| Tail power law exponent with p | γ3 = | 1.691 00
|
| p-value | p = | 0.000 00
|
| Outdegree tail power law exponent with p | γ3,o = | 1.711 00
|
| Outdegree p-value | po = | 0.000 00
|
| Indegree tail power law exponent with p | γ3,i = | 1.741 00
|
| Indegree p-value | pi = | 0.000 00
|
| Degree assortativity | ρ = | −0.040 645 7
|
| Degree assortativity p-value | pρ = | 9.654 33 × 10−294
|
| In/outdegree correlation | ρ± = | +0.736 595
|
| Clustering coefficient | c = | 0.065 678 8
|
| Directed clustering coefficient | c± = | 0.090 296 9
|
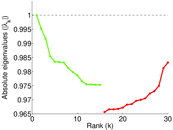
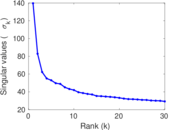
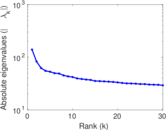
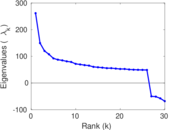
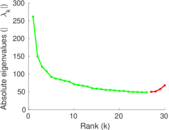
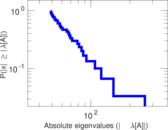
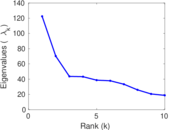
| Spectral norm | α = | 245.999
|
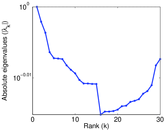
| Operator 2-norm | ν = | 139.616
|
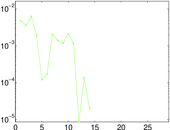
| Cyclic eigenvalue | π = | 106.528
|
| Algebraic connectivity | a = | 0.022 466 4
|
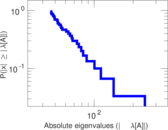
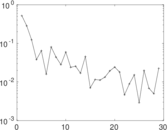
| Spectral separation | |λ1[A] / λ2[A]| = | 1.743 14
|
| Reciprocity | y = | 0.405 226
|
| Non-bipartivity | bA = | 0.723 602
|
| Normalized non-bipartivity | bN = | 0.016 785 3
|
| Algebraic non-bipartivity | χ = | 0.032 250 1
|
| Spectral bipartite frustration | bK = | 0.000 753 884
|
| Controllability | C = | 41,816
|
| Relative controllability | Cr = | 0.551 088
|
Plots

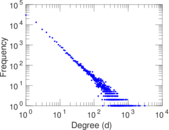
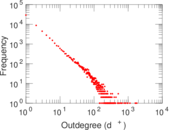
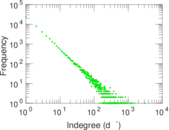
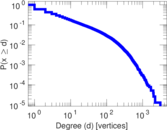


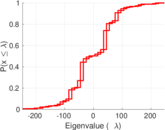
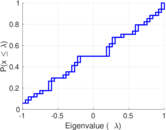
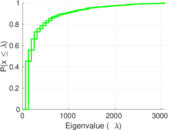

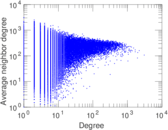


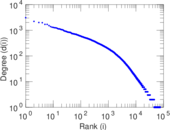
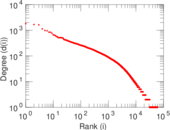
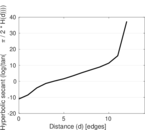

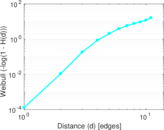
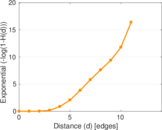




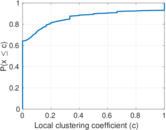


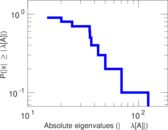
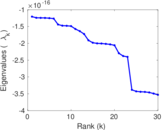
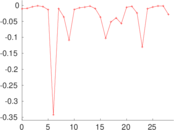
Matrix decompositions plots
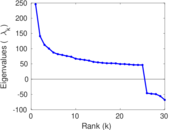
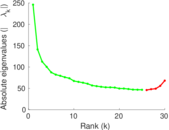
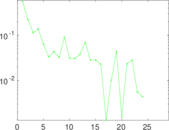
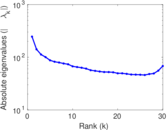

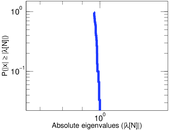
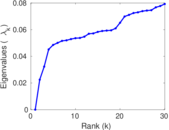
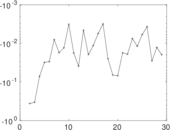
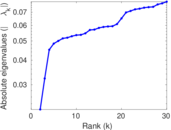

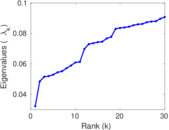
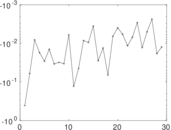
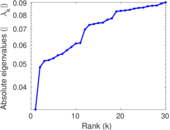
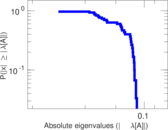
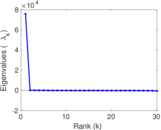
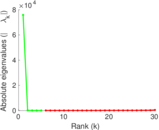
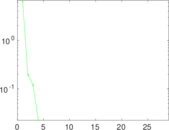
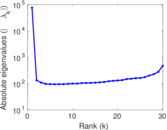

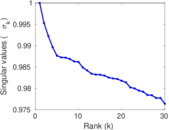
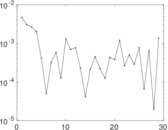
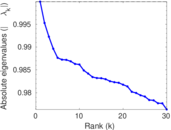
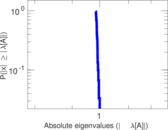
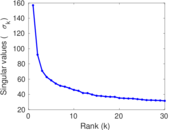
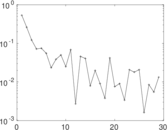
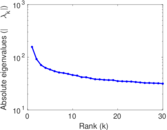
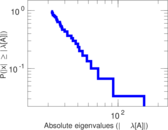
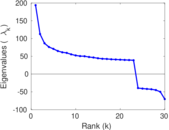

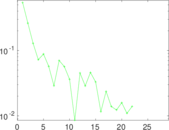

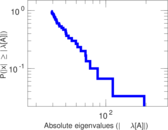
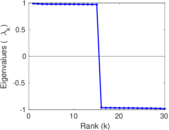

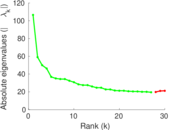
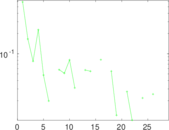

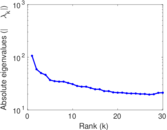
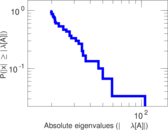
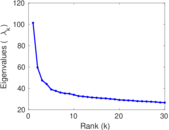
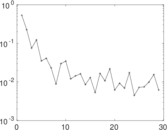
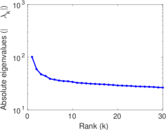
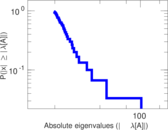
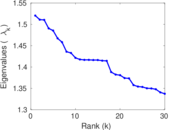
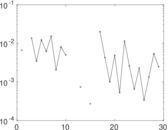

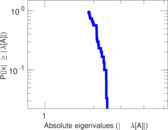
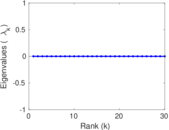


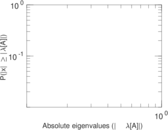



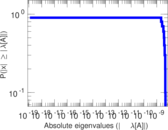

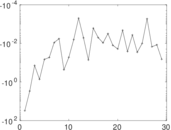

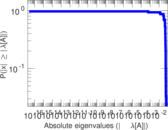
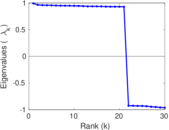
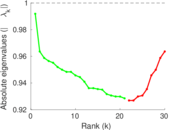
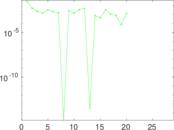


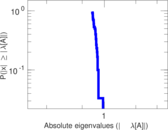
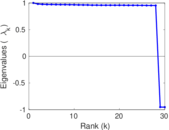


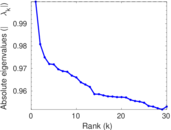
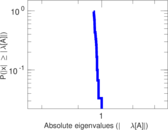
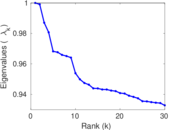
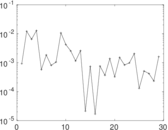
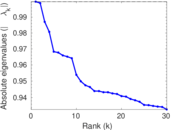
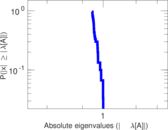




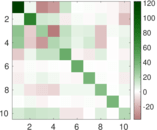
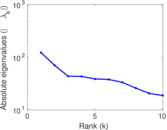
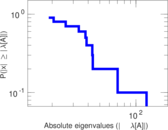
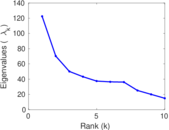



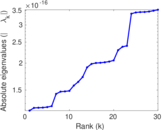
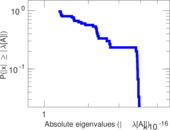
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Matthew Richardson, Rakesh Agrawal, and Pedro Domingos.
Trust management for the semantic web.
In Proc. Int. Semant. Web Conf., pages 351–368. 2003.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee
















































































































































