 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee
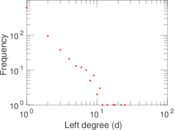
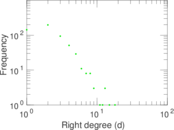
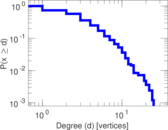
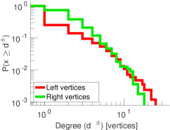
This bipartite network contains persons who appeared in at least one crime case as either a suspect, a victim, a witness or both a suspect and victim at the same time. A left node represents a person and a right node represents a crime. An edge between two nodes shows that the left node was involved in the crime represented by the right node.
| Code | MC
| |
| Internal name | moreno_crime
| |
| Name | Crime | |
| Data source | http://moreno.ss.uci.edu/data.html#crime | |
| Availability | Dataset is available for download | |
| Consistency check | Dataset passed all tests | |
| Category | Interaction network | |
| Node meaning | Person, crime | |
| Edge meaning | Involvment | |
| Network format | Bipartite, undirected | |
| Edge type | Unweighted, no multiple edges |




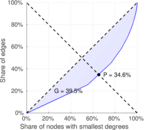
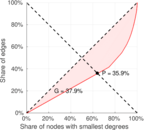
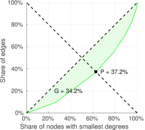
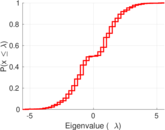
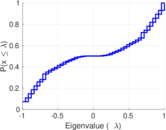
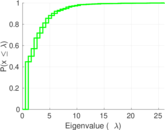



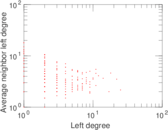
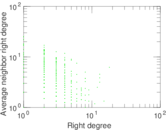
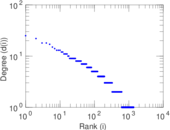
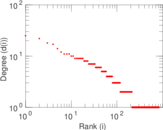
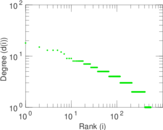
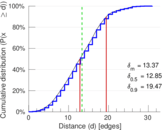
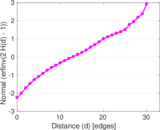
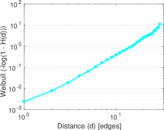
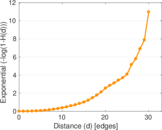

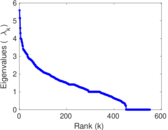
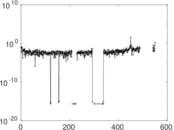

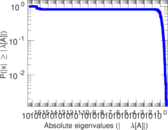
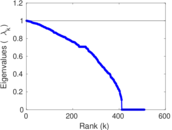
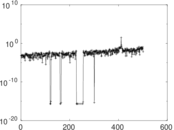
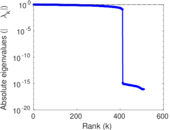


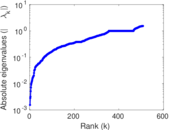
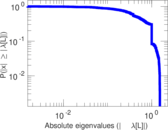
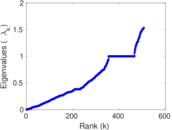

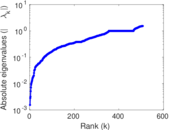
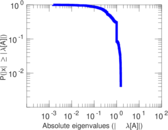
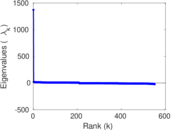
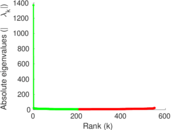
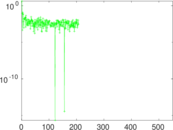

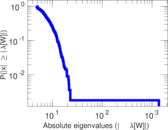
| [1] | Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ] |