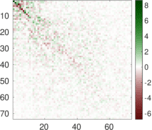
Congress votes
In this network, nodes are politicians speaking in the United States Congress,
and a directed edge denotes that a speaker mentions another speaker. The
weight of an edge (positive or negative) denotes whether the mention is in
support of or opposition to the mentioned politician. Multiple parallel edges
are possible. Loops are allowed, i.e., speakers may mention themselves.
Metadata
Statistics
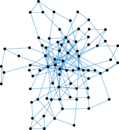
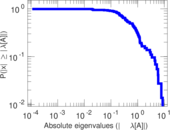
Plots


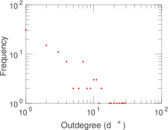
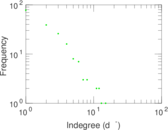
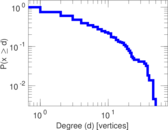

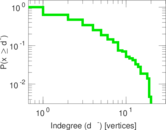
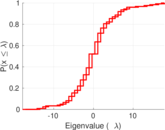
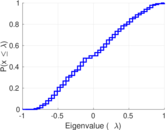
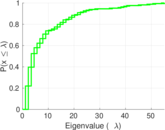


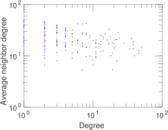
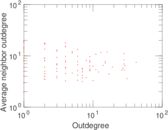
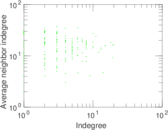



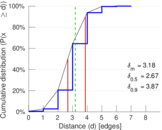
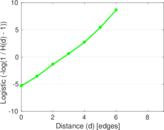
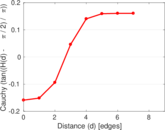

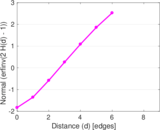
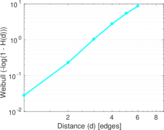
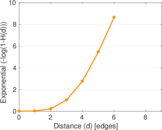


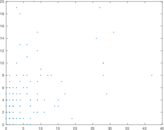
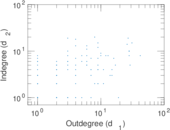


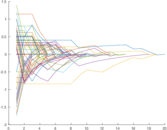
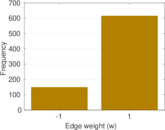
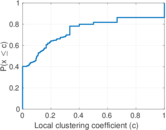
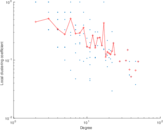
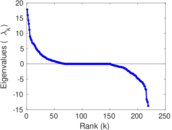
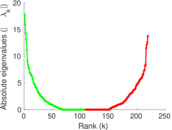
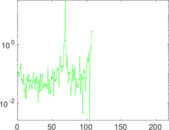
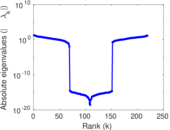
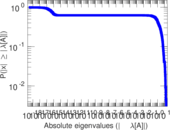
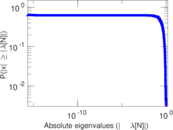
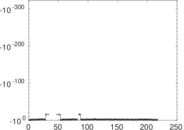
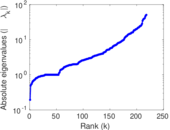
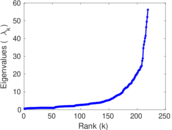
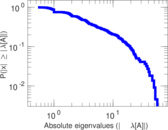
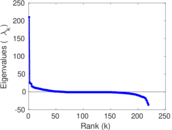
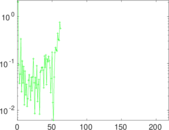
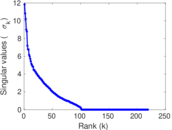
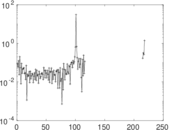
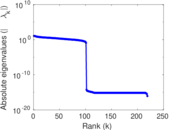
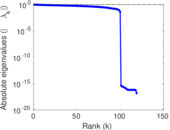
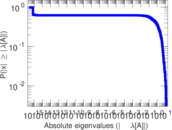
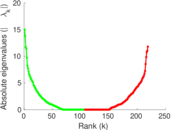
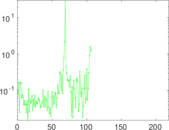
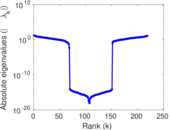
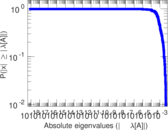
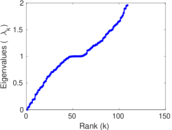
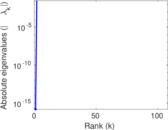
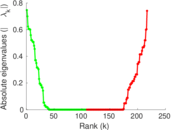
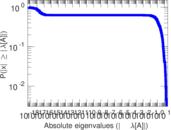
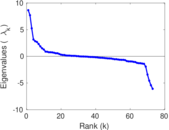
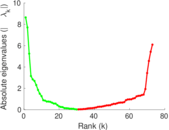
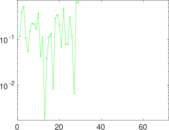
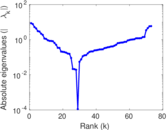
Matrix decompositions plots
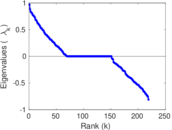
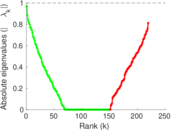
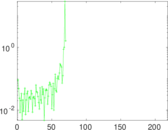
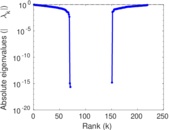









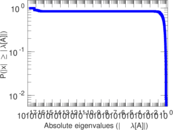
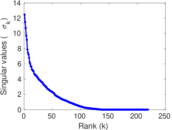
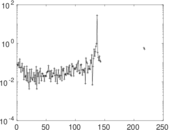
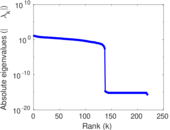

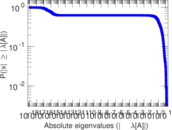
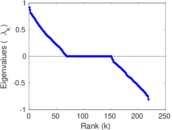
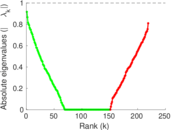

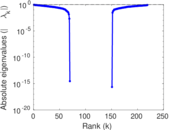
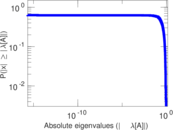

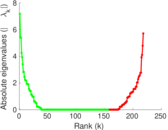

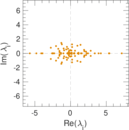
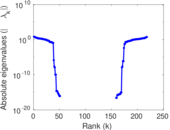
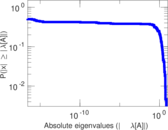
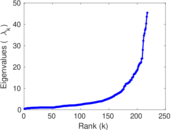
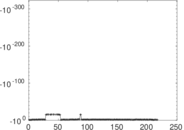
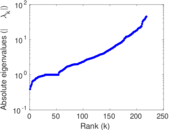


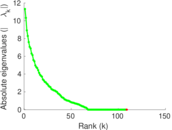
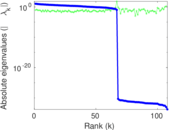
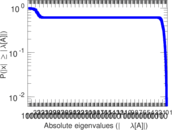
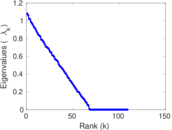
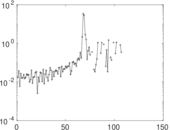
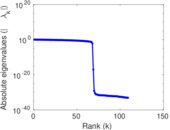
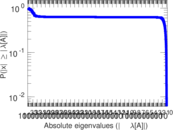

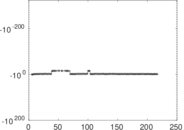
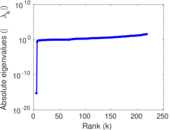
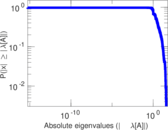
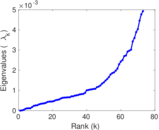
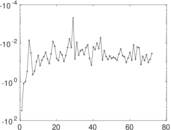




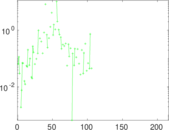

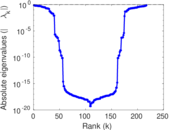

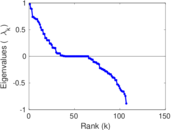
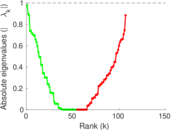
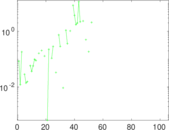

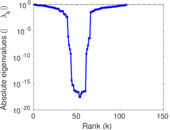


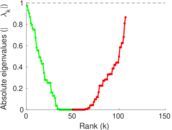
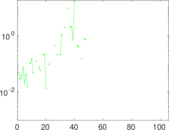
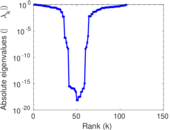

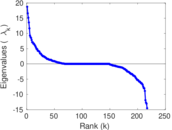
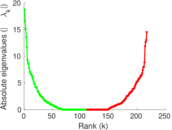


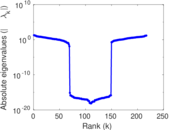


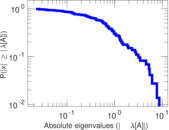
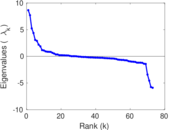
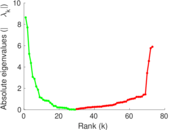
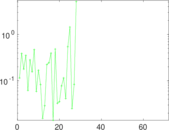
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Matt Thomas, Bo Pang, and Lillian Lee.
Get the out vote: Determining support or opposition from
congressional floor-debate transcripts.
In Proc. Conf. on Empir. Methods in Nat. Lang. Process., pages
327–335, 2006.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee

























































































































































