Wikiversity edits (de)
This is the bipartite edit network of the German Wikiversity. It contains users
and pages from the German Wikiversity, connected by edit events. Each edge
represents an edit. The dataset includes the timestamp of each edit.
Metadata
Statistics
| Size | n = | 82,453
|
| Left size | n1 = | 5,613
|
| Right size | n2 = | 76,840
|
| Volume | m = | 422,143
|
| Unique edge count | m̿ = | 117,865
|
| Wedge count | s = | 934,708,643
|
| Claw count | z = | 10,924,714,907,700
|
| Cross count | x = | 103,673,450,186,756,976
|
| Square count | q = | 44,229,078
|
| 4-Tour count | T4 = | 4,092,917,374
|
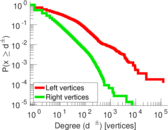
| Maximum degree | dmax = | 122,179
|
| Maximum left degree | d1max = | 122,179
|
| Maximum right degree | d2max = | 7,220
|
| Average degree | d = | 10.239 6
|
| Average left degree | d1 = | 75.208 1
|
| Average right degree | d2 = | 5.493 79
|
| Fill | p = | 0.000 273 277
|
| Average edge multiplicity | m̃ = | 3.581 58
|
| Size of LCC | N = | 80,278
|
| Diameter | δ = | 16
|
| 50-Percentile effective diameter | δ0.5 = | 3.467 73
|
| 90-Percentile effective diameter | δ0.9 = | 5.360 62
|
| Median distance | δM = | 4
|
| Mean distance | δm = | 3.903 88
|
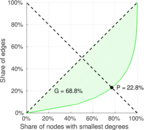
| Gini coefficient | G = | 0.827 823
|
| Balanced inequality ratio | P = | 0.158 362
|
| Left balanced inequality ratio | P1 = | 0.108 442
|
| Right balanced inequality ratio | P2 = | 0.228 389
|
| Relative edge distribution entropy | Her = | 0.723 801
|
| Power law exponent | γ = | 4.317 88
|
| Tail power law exponent | γt = | 2.961 00
|
| Tail power law exponent with p | γ3 = | 2.961 00
|
| p-value | p = | 0.000 00
|
| Left tail power law exponent with p | γ3,1 = | 1.941 00
|
| Left p-value | p1 = | 0.000 00
|
| Right tail power law exponent with p | γ3,2 = | 2.751 00
|
| Right p-value | p2 = | 0.484 000
|
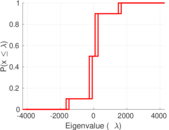
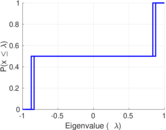
| Degree assortativity | ρ = | −0.141 225
|
| Degree assortativity p-value | pρ = | 0.000 00
|
| Spectral norm | α = | 4,282.78
|
| Algebraic connectivity | a = | 0.010 555 9
|
| Spectral separation | |λ1[A] / λ2[A]| = | 2.487 18
|
| Controllability | C = | 73,589
|
| Relative controllability | Cr = | 0.896 738
|
Plots


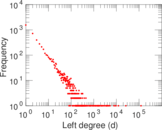
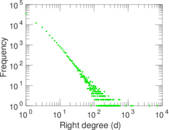
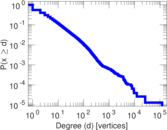

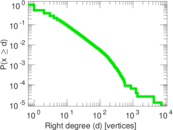















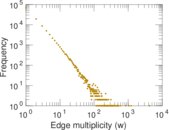

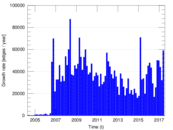

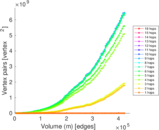
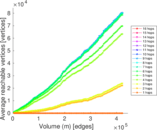

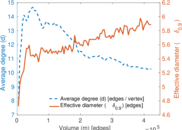
Matrix decompositions plots
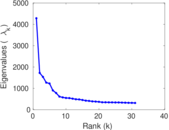
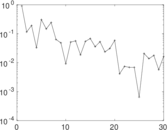
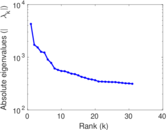
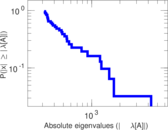
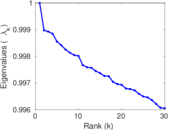

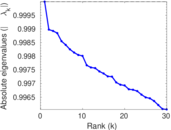
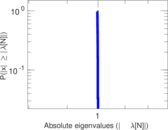
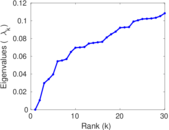
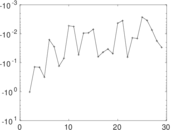

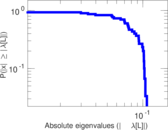
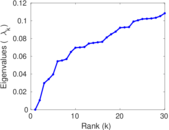
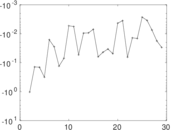
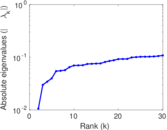
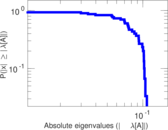

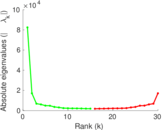

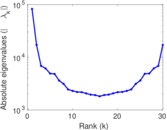

Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Wikimedia Foundation.
Wikimedia downloads.
http://dumps.wikimedia.org/, January 2010.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee































































