Premier League (2013/2014)

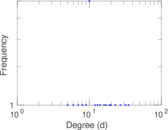
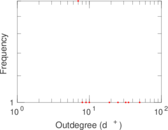
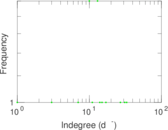
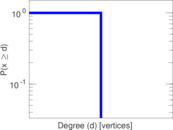
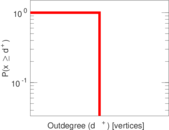
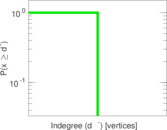
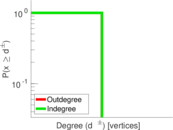
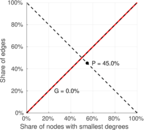
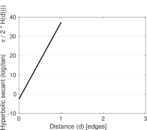
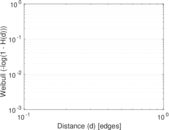
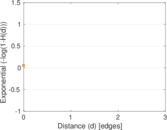
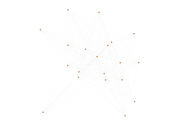
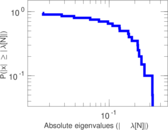
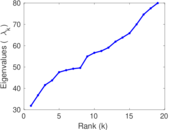
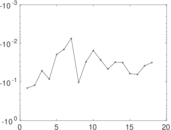
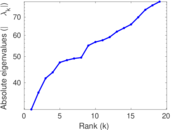
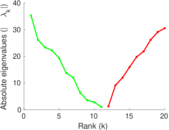
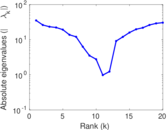
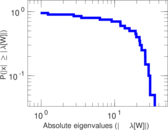
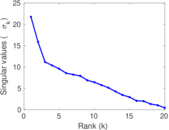
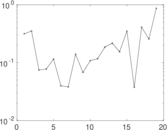
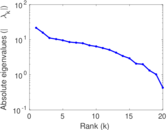
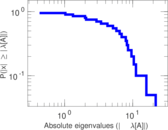
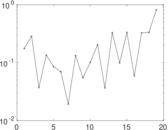
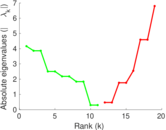
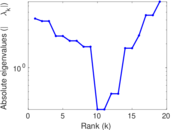
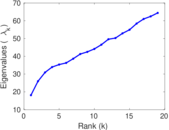
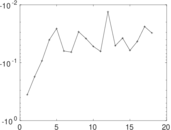
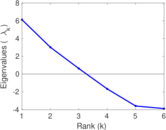
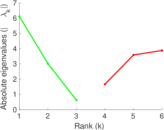
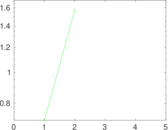
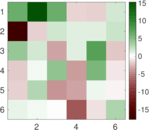
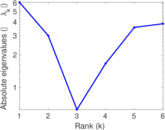
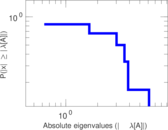
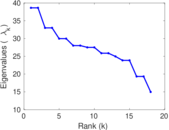
These are results of football games in England and Wales from the Premier League in the season 2013/2014, in form of a directed, signed graph. Nodes are teams, and each directed edge from A to B denotes that team A played at home against team B. The edge weights are the goal difference, and thus positive if the home team wins, negative when the away team wins, and zero for a draw. The exact game results are not represented; only the goal differences are. The data was copied by hand from Wikipedia.

Metadata

Codeu3
Internal nameleague-uk1-2013
NamePremier League (2013/2014)
AvailabilityDataset is available for download
Consistency checkDataset passed all tests
Category
Interaction network
Dataset timestamp 2013 ⋯ 2014
Node meaningTeam
Edge meaningGame
Network formatUnipartite, directed
Edge typeSigned, possibly weighted, multiple edges
ReciprocalContains reciprocal edges
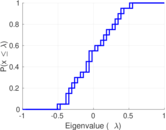
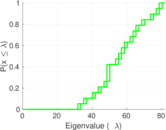
Directed cyclesContains directed cycles
LoopsDoes not contain loops
Skew-symmetry Inverted edges can be interpreted as negated edges
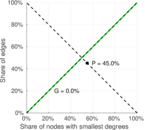
Complete Edges exist between all possible nodes
Zero weights Edges may have weight zero

Statistics

Size n =20
Volume m =380
Unique edge count m̿ =302
Loop count l =0
Wedge count s =3,420
Claw count z =84,608
Cross count x =587,190
Triangle count t =1,140
Square count q =12,029
4-Tour count T4 =109,026
Maximum degree dmax =38
Maximum outdegree d+max =19
Maximum indegree d−max =19
Average degree d =38.000 0
Fill p =0.794 737
Average edge multiplicity m̃ =1.258 28
Size of LCC N =20
Size of LSCC Ns =20
Relative size of LSCC Nrs =1.000 00
Diameter δ =1
50-Percentile effective diameter δ0.5 =0.473 333
90-Percentile effective diameter δ0.9 =0.894 667
Median distance δM =1
Mean distance δm =0.949 367
Gini coefficient G =0.043 211 9
Balanced inequality ratio P =0.470 199
Outdegree balanced inequality ratio P+ =0.456 954
Indegree balanced inequality ratio P− =0.466 887
Relative edge distribution entropy Her =0.998 852
Power law exponent γ =6.383 36
Tail power law exponent γt =8.991 00
Tail power law exponent with p γ3 =8.991 00
p-value p =0.000 00
Outdegree tail power law exponent with p γ3,o =6.021 00
Outdegree p-value po =0.000 00
Indegree tail power law exponent with p γ3,i =8.991 00
Indegree p-value pi =0.178 000
Degree assortativity ρ =−0.095 939 0
Degree assortativity p-value pρ =0.068 264 1
In/outdegree correlation ρ± =+0.251 548
Clustering coefficient c =1.000 00
Directed clustering coefficient c± =0.791 359
Spectral norm α =19.426 8
Operator 2-norm ν =21.744 5
Cyclic eigenvalue π =2.507 21
Algebraic connectivity a =36.750 8
Spectral separation |λ1[A] / λ2[A]| =1.085 24
Reciprocity y =0.801 325
Non-bipartivity bA =0.795 621
Normalized non-bipartivity bN =0.818 136
Algebraic non-bipartivity χ =13.167 0
Spectral bipartite frustration bK =0.181 865
Negativity ζ =0.407 285
Algebraic conflict ξ =19.954 3
Triadic conflict τ =0.511 146
Spectral signed frustration φ =0.493 919
Controllability C =0
Relative controllability Cr =0.000 00

Plots

Fruchterman–Reingold graph drawing

Degree distribution

Cumulative degree distribution

Lorenz curve

Spectral distribution of the adjacency matrix

Spectral distribution of the normalized adjacency matrix

Spectral distribution of the Laplacian

Spectral graph drawing based on the adjacency matrix

Spectral graph drawing based on the Laplacian

Spectral graph drawing based on the normalized adjacency matrix

Degree assortativity

Zipf plot

Hop distribution

Double Laplacian graph drawing

Delaunay graph drawing

In/outdegree scatter plot

Item rating evolution

Edge weight/multiplicity distribution

Clustering coefficient distribution

Average neighbor degree distribution

SynGraphy

Matrix decompositions plots

Downloads

References

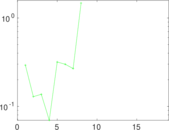
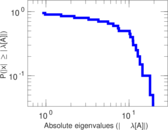
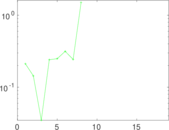
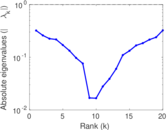
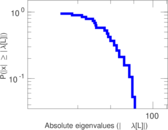
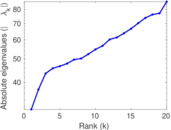
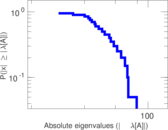
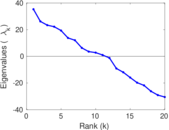
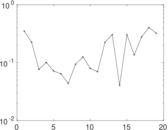
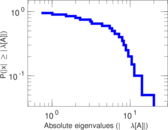
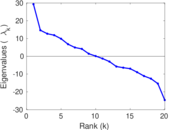
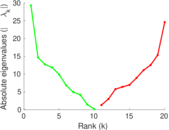
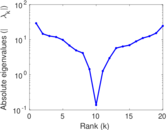
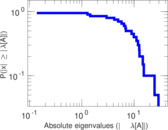
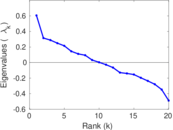
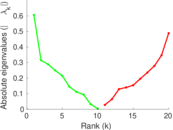
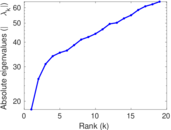
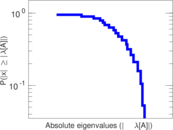
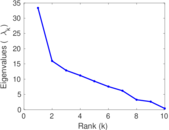
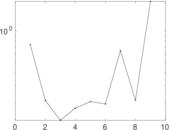
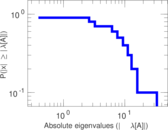
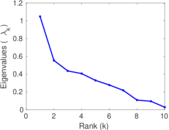
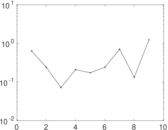
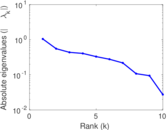
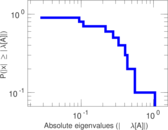
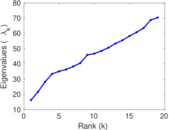
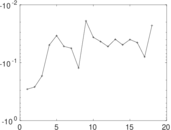
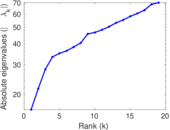
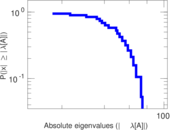
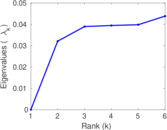
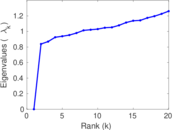
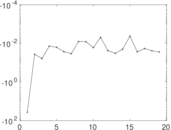
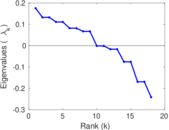
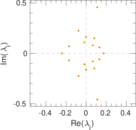
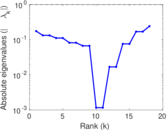
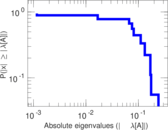
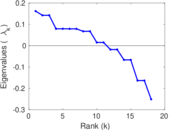
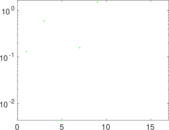
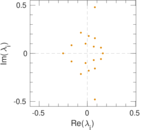
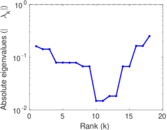
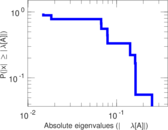
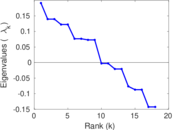
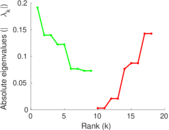
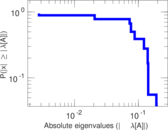
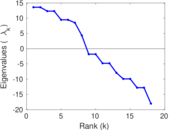
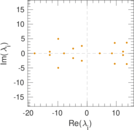
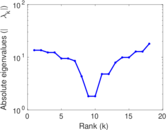
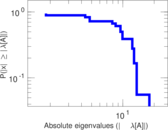
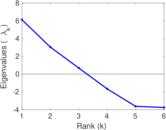
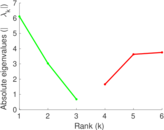
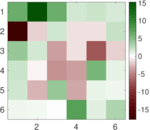
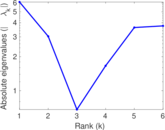
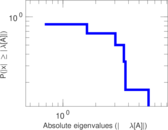
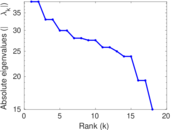
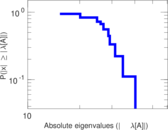
[1] Jérôme Kunegis. KONECT – The Koblenz Network Collection. In Proc. Int. Conf. on World Wide Web Companion, pages 1343–1350, 2013. [ http ]
[2] Michaël Fanuel and Johan A. K. Suykens. Deformed Laplacians and spectral ranking in directed networks. Applied and Computational Harmonic Analysis, 2017. In press.