Twitter mentions
This is the directed network of "@username" mentions on Twitter. Each node is a
Twitter user, and each directed edge from user A to user B means that user A
has mentioned user B in a tweet using the "@username" syntax. Multiple edges
are allowed, and each edge is annotated with the timestamp of the tweet. Since
it is possible to mention one's own username, this network contains loops.
Metadata
Statistics
| Size | n = | 2,919,613
|
| Volume | m = | 12,887,063
|
| Unique edge count | m̿ = | 7,301,101
|
| Loop count | l = | 235,300
|
| Wedge count | s = | 736,717,625
|
| Claw count | z = | 690,658,257,498
|
| Cross count | x = | 1,640,036,248,049,757
|
| Triangle count | t = | 1,449,797
|
| Square count | q = | 81,161,696
|
| 4-Tour count | T4 = | 3,610,479,352
|
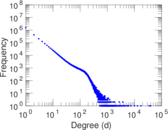
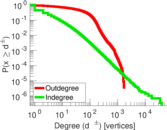
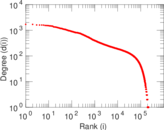
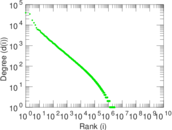
| Maximum degree | dmax = | 39,753
|
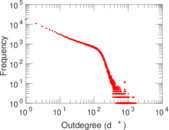
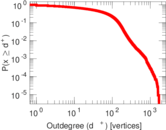
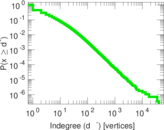
| Maximum outdegree | d+max = | 1,697
|
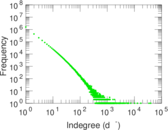
| Maximum indegree | d−max = | 39,753
|
| Average degree | d = | 8.827 93
|
| Fill | p = | 8.565 21 × 10−7
|
| Average edge multiplicity | m̃ = | 1.765 08
|
| Size of LCC | N = | 2,893,623
|
| Size of LSCC | Ns = | 98,784
|
| Relative size of LSCC | Nrs = | 0.033 834 6
|
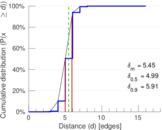
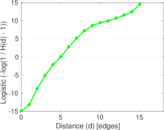
| Diameter | δ = | 23
|
| 50-Percentile effective diameter | δ0.5 = | 4.987 02
|
| 90-Percentile effective diameter | δ0.9 = | 5.908 45
|
| Median distance | δM = | 5
|
| Mean distance | δm = | 5.454 36
|
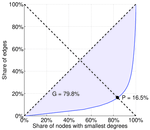
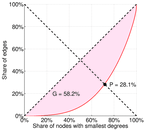
| Gini coefficient | G = | 0.798 378
|
| Balanced inequality ratio | P = | 0.164 585
|
| Outdegree balanced inequality ratio | P+ = | 0.281 465
|
| Indegree balanced inequality ratio | P− = | 0.229 310
|
| Relative edge distribution entropy | Her = | 0.894 306
|
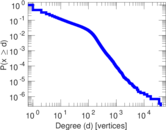
| Power law exponent | γ = | 2.845 35
|
| Tail power law exponent | γt = | 1.861 00
|
| Degree assortativity | ρ = | −0.010 567 6
|
| Degree assortativity p-value | pρ = | 0.000 00
|
| Clustering coefficient | c = | 0.005 903 74
|
| Directed clustering coefficient | c± = | 0.025 903 2
|
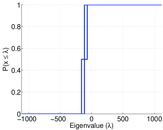
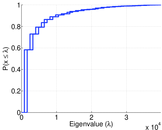
| Spectral norm | α = | 1,114.49
|
| Operator 2-norm | ν = | 1,112.63
|
| Cyclic eigenvalue | π = | 345.070
|
| Spectral separation | |λ1[A] / λ2[A]| = | 1.002 88
|
| Reciprocity | y = | 0.031 959 8
|
| Non-bipartivity | bA = | 0.002 874 42
|
| Normalized non-bipartivity | bN = | 0.000 598 655
|
| Algebraic non-bipartivity | χ = | 0.001 197 18
|
| Spectral bipartite frustration | bK = | 6.019 94 × 10−5
|
| Controllability | C = | 2,710,455
|
| Relative controllability | Cr = | 0.928 361
|
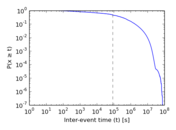
Plots



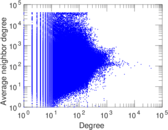
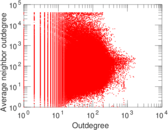
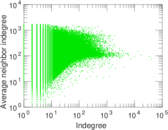
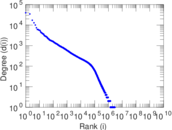
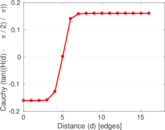


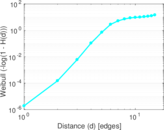
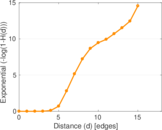
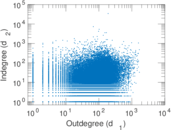

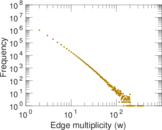

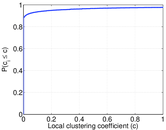
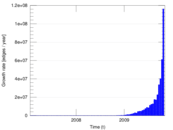

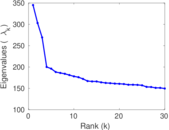
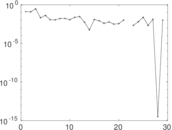
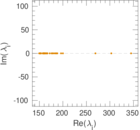
Matrix decompositions plots
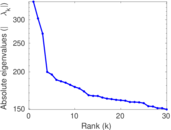
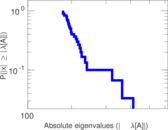

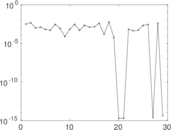
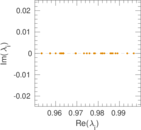
Downloads
References
|
[1]
|
Jérôme Kunegis.
KONECT – The Koblenz Network Collection.
In Proc. Int. Conf. on World Wide Web Companion, pages
1343–1350, 2013.
[ http ]
|
|
[2]
|
Munmun De Choudhury, Yu-Ru Lin, Hari Sundaram, K. Selçuk Candan, Lexing Xie,
and Aisling Kelliher.
How does the data sampling strategy impact the discovery of
information diffusion in social media?
In ICWSM, pages 34–41, 2010.
|
 KONECT ‣ Networks ‣
Buy Me a Coffee
KONECT ‣ Networks ‣
Buy Me a Coffee


















































